?> Options Trading Archives - Page 12 of 17 - projectfinance

Category: Options Trading

Iron Condors vs. Strangles: Profit/Loss Analysis

Posted on by Chris Butler

Last updated on February 10th, 2022 , 07:22 pm

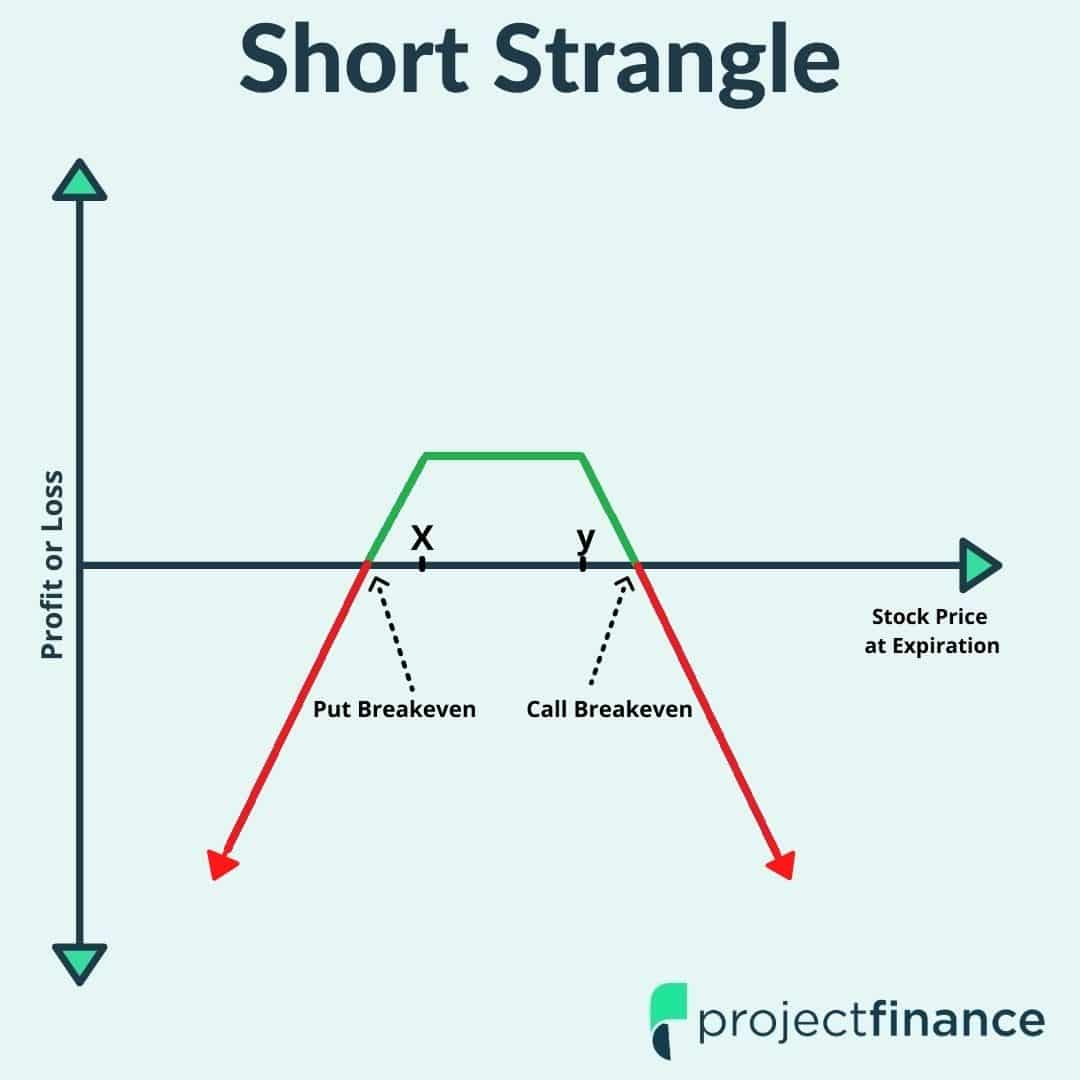
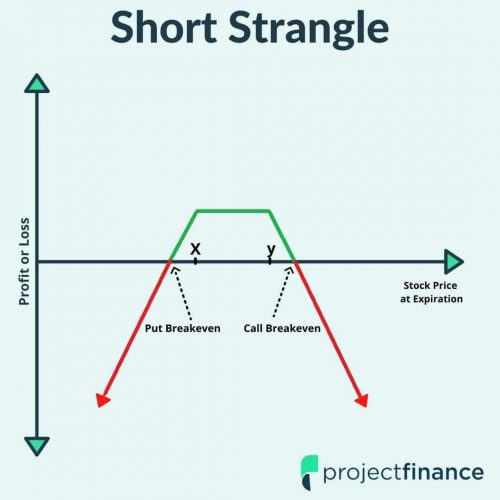
Short iron condors and short strangles are very common strategies among market-neutral traders, as both strategies profit from range-bound stock price movements.

However, iron condors have less risk (and therefore less reward), while strangles have more risk and more reward.

How much reward do you give up when selling an iron condor instead of a short strangle?

In this post, we’ll compare iron condors vs. strangles in regards to historical profits and losses in the S&P 500.

Study Methodology: 16-Delta Short Options

For our iron condors vs. strangles study, we used the following methodology to maximize the number of trades tested:

Underlying: S&P 500 ETF (SPY) from 2007 to Present

Entry Dates: Every Trading Day

Target Time to Expiration: 60 Days

Trade #1: Short 16-Delta Strangle (Short 16-Delta Call; Short 16-Delta Put)

Trade #2: Short Iron Condor (16-Delta Short Calls & Puts; 5-Delta Long Calls & Puts)

Trade #3: Short Iron Condor (16-Delta Short Calls & Puts; 10-Delta Long Calls & Puts)

We entered each of the above positions on every trading day and held the positions to expiration. Of course, a new trade wouldn’t be entered every single trading day, but by conducting our study this way, we remove the sensitivity of the start date from the results.

Finally, we summed the cumulative profit/loss of each approach to visualize the performance.

Here were the results:

 

Iron Condors vs. Strangles: SPY 16-Delta

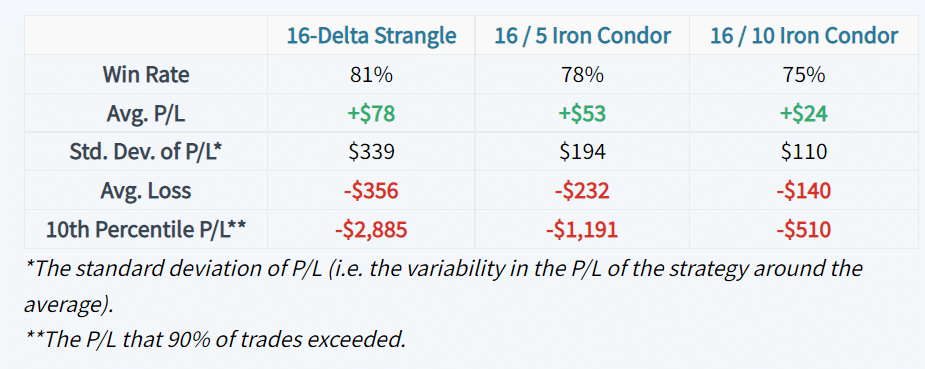
To clarify, a “16-Delta / 5-Delta Iron Condor” indicates 16-delta short calls and puts with 5-delta long calls and puts.

As we might expect, the short strangles performed the best. Since the iron condor positions purchase out-of-the-money options against the short options, the net premium received is lower, which reduces profit potential.

At the same time, the short strangle approach had the most substantial drawdown in 2008, as strangles have no protection.

Furthermore, the strategy with the least volatility and profitability was the iron condor approach that purchased 10-delta options agains the 16-delta short options. Understandably, this approach had the “smoothest” path, as the strategy has the least profit and loss potential because the long options were much closer to the short options.

Iron Condors vs. Strangles By the Numbers

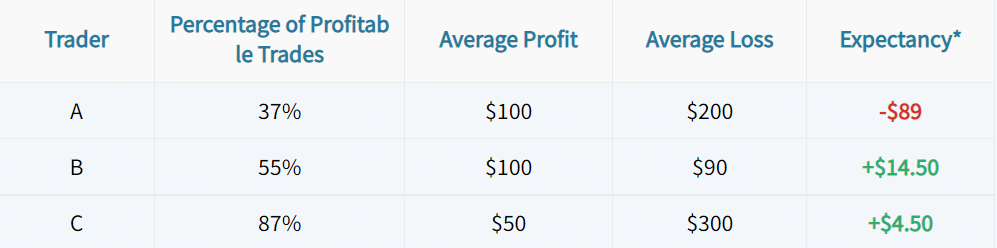
Let’s take a look at some metrics related to each approach:

 

iron condor vs strangle

By analyzing these metrics, we confirm our previous statements about the average profitability of each approach.

Interestingly, the 16 / 5 iron condor variation did not suffer too significant of a win rate or average P/L decrease, but the 10th percentile P/L was substantially better. In other words, limiting the loss potential on a short strangle by purchasing 5-delta calls and puts will reduce profit potential and the rate of success, but will help avoid catastrophic losses that strangles will suffer from during “black swan” market events.

Let’s do the same test on 30-delta iron condors and strangles.

Study Methodology: 30-Delta Short Options

Underlying: S&P 500 ETF (SPY) from 2007 to Present

Entry Dates: Every Trading Day

Target Time to Expiration: 60 Days

Trade #1: Short 30-Delta Strangle (Short 30-Delta Call; Short 30-Delta Put)

Trade #2: Short Iron Condor (30-Delta Short Calls & Puts; 16-Delta Long Calls & Puts)

Trade #3: Short Iron Condor (30-Delta Short Calls & Puts; 10-Delta Long Calls & Puts)

Like the previous test, all trades were held to expiration, and the expiration P/L for each trade was summed over the test period.

Here were the results:

Iron Condors vs. Strangles: SPY 30-Delta

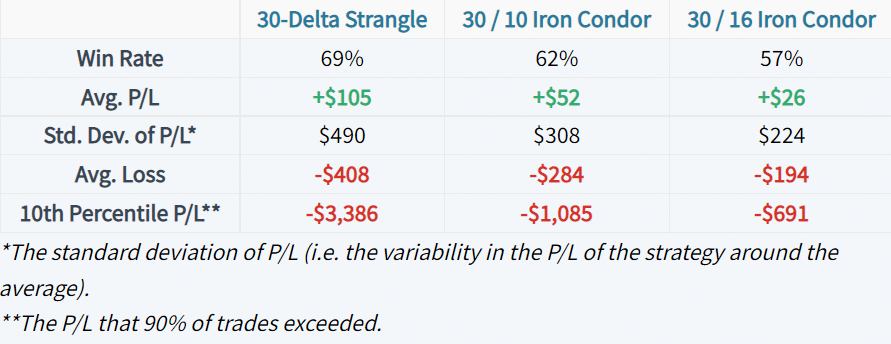
Consistent with the previous iron condor and strangle variations, the strangles had the largest drawdowns and the highest overall P/L. Additionally, the 30 / 16 iron condor variation was much less risky, and therefore less rewarding than the 30 / 10 iron condor.

Iron Condors vs. Strangles By the Numbers

Here are the profitability metrics related to each approach:

condor vs strangle

Consistent with previous findings, purchasing closer options against the short strangles (therefore reducing the maximum profit potential and risk relative to the short strangle) reduced the percentage of profitable trades, and the average profitability.

However, compared to the short strangles, the iron condor approaches had notably better loss metrics.

Implied Volatility at Entry

How did all of these approaches perform when implied volatility was low or high at the time of trade entry?

To run this test, we equally divided all of the trades into two buckets based on the VIX Index at entry. Our threshold for the dividing line between high and low IV is 17.5, as that is the median VIX closing price over the period.

To run this test, we equally divided all of the trades into two buckets based on the VIX Index at entry. Our threshold for the dividing line between high and low IV is 17.5, as that is the median VIX closing price over the period.

Win Rates: High & Low IV

Let’s start by analyzing any changes in the percentage of profitable trades in the high vs. low IV entries:

Interestingly, the strategies with further short options (16-delta) realized a slightly higher percentage of profitable trades in the “low” implied volatility entries, while the 30-delta strategies didn’t see changes.

Average Profit/Loss: High & Low IV

Let’s look at the average P/L per trade for each approach in the high and low implied volatility entries.

We’ll start with the 16-delta iron condors and strangles:

 

Iron Condors vs. Strangles: Average P/L 16-Delta

And now the 30-delta iron condors and strangles:

 

Iron Condors vs. Strangles: Average P/L 30-Delta

In both the 16-delta and 30-delta short strangle setups, the trades experienced higher average P/L with the lower implied volatility entries.

In regards to the 16-delta iron condors, the results were somewhat similar. However, the 30-delta iron condor setups experienced higher average P/L with the higher implied volatility entries.

To understand where the differences are coming from, let’s analyze the worst-case losses for each approach.

10th Percentile P/L: High & Low IV

The 10th percentile P/L tells us the P/L figure that 90% of trades exceeded. In other words, only 10% of trades had a P/L lower than the 10th percentile P/L, which gives us an idea of the “outlier” returns for each strategy.

Let’s start with the 16-delta approaches:

 

Iron Condors vs. Strangles: 10th Percentile P/L

As we can see, the short strangles experienced substantially larger “worst-case” losses in the high implied volatility entries. The difference was less substantial for the iron condor setups.

Let’s see if we find the same relationship in the 30-delta setups:

 

Iron Condors vs. Strangles: 10th Percentile P/L

Consistent with previous findings, the short strangles entered in lower implied volatility environments realized substantially lower “worst-case” drawdowns than the high IV short strangles.

The data from the loss metrics suggest that the improvement in the average P/L for the lower implied volatility entries (regarding the short strangles in particular) stems from less severe drawdowns.

Summary of Main Concepts

Here are the primary findings from this iron condor vs. strangle analysis:

 

•As we’d expect, the average profitability of iron condors tends to be lower than a short strangle approach over time, as buying protection in the form of long calls and puts against short strangles reduces the profit potential.

 

•However, by limiting the loss potential (selling an iron condor instead of a strangle), the drawdowns are much less severe, which can improve average profitability over time.

 

•When filtering the trades for high vs. low implied volatility entries, we saw a substantial improvement in the average profitability of the 16-delta and 30-delta short strangles that were sold in the lower implied volatility environment.

 

•When filtering the iron condor trades for low and high IV entries, the differences were much more muted compared to the differences observed in the short strangles.

 

•The findings do not “crown” any of the studied strategies as the definite winners, but we do learn that selling uncovered options in high implied volatility environments can be very risky, as high implied volatility typically occurs when market fluctuations are substantial (high levels of historical volatility).

 

•In the same vein, traders should be very careful when selling uncovered options in lower implied volatility environments, as the transition into a high implied volatility environment with substantial realized market movements can lead to significant drawdowns.

Next Lesson

Additional Resources

Iron Condor vs Strangles – Options Jive | tastytrade

Chris Butler portrait

9 Common Options Trading Mistakes – Don’t Do This!

Posted on by Mike Martin

Last updated on February 10th, 2022 , 07:24 pm

There are many options trading mistakes that new traders make, and that’s entirely ok! Part of the process when learning anything in life is through mistakes.

With that said, it’s always good to be aware of common “land mines,” so you can make an attempt to avoid them, and ultimately protect your hard-earned money.

In this post, we’ll cover our picks for the top nine options trading mistakes that most new traders will make at some point.

1. No Exit Plan

It’s crucial to know exactly when you will close a trade, profitable or unprofitable.

Perhaps the most important thing to know is when you’ll close losing trades that carry a lot of risk, such as being short naked options. When trading limited-risk spreads, it’s ok to be accepting of the maximum loss potential of that position, so long as you’ve sized the position accordingly.

2. Trading Too Many Positions

Some may not agree that this is a mistake, but the fact of the matter is that it’s more difficult to keep track of your portfolio when you trade more and more positions.

Additionally, it’s likely that your positions are highly correlated, which means you’re really trading similar positions in different stocks. It’s ok to have multiple positions in different stocks, just be aware that they may react the same way when markets get volatile.

Lastly, during extremely volatile trading sessions, getting filled on your trades is next to impossible. If you have on 20 positions, good luck making timely adjustments in those positions at decent prices.

The only caveat to this particular point is that if you’re a brand new trader, you’ll benefit from more positions because you’ll gain more experience in regards to strategies and trade outcomes.

3. Trading Illiquid Products

Getting trapped in an option position that will be impossible to close can be costly. If you enter a position in options that are illiquid (very little to no volume or open interest), then it’s likely you’ll be exiting that position at a very unfavorable price

At the very minimum, trade options with open interest in the 1,000s and volume in the 100s (though volume will be lower early in the trading session).

4. Trying to Fix Entirely Broken Trades

Trying to fix broken trades is another common options trading mistake. At some point, the trade is no longer worth your commissions, and you’d be better off taking the loss and allocating your capital elsewhere.

There will always be another trading opportunity, so don’t waste your time trying to repair something that has a very low probability of getting fixed.

5. Taking Profits Too Soon

While taking profits on a trade is not a bad thing, creating a strategy that revolves around taking tiny profits may seem logical at first, as your probability of success will be incredibly high (90% or higher in some cases).

However, you must keep in mind that such approaches require you to have a very high success rate, as the inevitable losses will likely consume a good portion of your profits from winning trades.

Furthermore, commissions will eat into your returns when you take small profits, so try and let your profits run a little longer. A recent iron condor study we conducted showed that the 25% profit target combinations resulted in the lowest profit expectancy over time (sometimes negative when adjusting for estimated commissions), despite having success rates over 90%.

Of course, the strategy you implement should also be considered. For example, long calendar spreads are one example of trades that are typically closed at profit targets of 10-20%, as they don’t often reach profit levels of 50-100% on the debit paid. Another strategy in which profits are typically taken sooner is the short straddle, as the probability of reaching 50-100% of the profit potential is typically low.

6. Trading Too Big

This list of trading mistakes wouldn’t be complete without mentioning trade size.

Trading involves losses, and you can’t allow one losing trade to take you out. As a general guideline, you shouldn’t risk more than 2.5% of your portfolio in a short-term option position. Of course, this may vary based on risk tolerance, and it will be harder to comply with in smaller trading accounts, but don’t put 50% of your portfolio into a single option position!

If you’re trading longer-term options as a way to get leveraged exposure (such as buying 1-2 year call options on an equity), you may be able to get away with increasing your allocation.

7. Not Analyzing Implied Volatility

Implied volatility can be used to assess how “expensive” or “cheap” option prices currently are.

You should always analyze implied volatility before entering a trade to ensure that you won’t be battling changes in IV if your stock price outlook turns out to be correct.

One example of this would be buying call options after a severe market downturn. Typically, when stocks fall considerably, option prices get bid up and implied volatility rises. If you buy a call option in anticipation of a market increase, you might not make money if the market doesn’t increase enough to offset the inevitable decrease in implied volatility.

8. Not Checking for Market Catalysts

It’s always a good idea to make sure you’re aware of any upcoming events that could cause your stock to shift substantially in either direction.

More specifically, if a stock’s implied volatility has been rising and seems lofty, it’s usually for a good reason.

In regards to individual equities, earnings announcements will often cause a stock’s option prices to decay more slowly, leading to an increase in implied volatility as the earnings date approaches.

After the market catalyst passes and the uncertainty dissipates, option prices will change accordingly, but the stock/market may change significantly in one direction.

Be aware of such events before entering trades.

9. Trading Complex Products Without Researching Them First

There are some complicated products out there, particularly in the leveraged ETF and volatility space. Don’t worry! It takes some time to grasp how some of these products work. Just be sure that you read about them before trading them, as it will help avoid unexpected outcomes.

As an example, don’t sell that put spread in VXX until you understand what drives its movements!

That wraps up our picks for common options trading mistakes (in our opinion)!

Next Lesson

Supplemental Reading

Five Mistakes to Avoid When Trading Options – Options Playbook

Chris Butler portrait

Short Strangle Adjustments: Rolling the Calls

Posted on by Chris Butler

Last updated on February 10th, 2022 , 07:27 pm

Short strangle chart

Sometimes, you’ll need to make an adjustment to your option positions when the stock price moves against you.

In this post, we’re going to discuss the short strangle adjustment strategy of “rolling down” the short call options.

What is “Rolling an Option?”

Rolling an option is the process of closing an existing option and opening a new option at a different strike price or in a different expiration cycle.

Today, we’ll focus on “rolling down” the short call option in a short strangle position, which refers to buying back your current call option and “rolling it down” by selling a new call at a lower strike price.

When Do You Roll Down the Call?

Let’s talk about when a trader would most likely roll down the short call.

Consider the following visual:

 

Short Strangle Adjustments: Rolling Down the Short Calls

When the stock price falls quickly and approaches the short put’s strike price, the trade becomes directionally bullish. Why? Since short strangles have negative gamma, the position’s delta grows positive as the stock price trends towards the short put.

The result?

The trader starts to lose more and more money as the stock price continues to fall.

One potential short strangle adjustment a trader can make in this scenario is to roll down the short call options:

 

Short Strangle Adjustments: Rolling Down the Short Calls

To roll down the short call option, a trader simply has to buy back their current short call option and sell a new call option at a lower strike price (in the same expiration cycle).

What Does Rolling Down the Calls Accomplish?

By rolling down the short call option in a short strangle position, a trader accomplishes two things:

1. Collect more option premium since the new call you sell is more expensive than the call you buy back.

2. Your position’s delta becomes more neutral, which means you’ll lose less money if the stock price continues to decrease.

Let’s cover each of these points in more depth.

#1: Collect More Option Premium

Consider a trader who is short the 250 call in their short strangle position but rolls down to the 240 call:

delta options pic

Since the trader buys back the 250 call for $0.11 and sells the 240 call for $2.50, they collect more option premium from the roll.

Premium Collected:

$2.50 Collected – $0.11 Paid Out = +$2.39

In dollar terms, the additional $2.39 in premium means the maximum profit on the trade increases by $239 per short strangle, and the lower breakeven point is also pushed $2.39 lower.

As a result, the stock price can fall even further than it could before and the trade can still be profitable.

#2: Neutralize Your Position Delta

By rolling down the call option, the position also becomes more neutral.

Let’s say that at the time of the roll, the short strangle’s position delta is +45 (the trader is expected to lose $45 from a $1 decrease in the stock price, and make $45 from a $1 increase in the stock price).

Here’s how the position delta would change after the rolling adjustment from the previous example:

delta

Old Call Position Delta: -5 (+0.05 Call Delta x $100 Option Multiplier x -1 Contract)

New Call Position Delta: -47 (+0.47 Call Delta x $100 Option Multiplier x -1 Contract)

Change in Position Delta: -42

New Short Strangle Position Delta: +45 – 42 = +3

After rolling down the short call, the position delta becomes more neutral.

With a new position delta of +3, the trader is only expected to lose $3 if the stock price decreases by $1, as opposed to a $45 loss before the roll.

Of course, this also means the trader is only expected to gain $3 from a $1 increase in the share price, as opposed to a $45 gain before the short strangle adjustment.

With that said, it’s clear that there are some downsides to rolling down the short call.

What’s the Risk of Rolling Down the Short Calls?

While rolling down the short call increases the option premium received (higher maximum profit potential) and neutralizes your position delta, there are some downsides:

1. You decrease the range of maximum profitability, as your new call’s strike price is much closer to the short put’s strike price (and the maximum profit zone for a short strangle is the area in-between the short call and short put strike prices).

2. The position delta gets neutralized, which means a subsequent increase in the stock price results in less profits than if the rolling adjustment wasn’t made. Additionally, the position delta will start to grow negative if the stock price continues to increase after rolling down the short call (resulting in losses if the stock keeps rising).

As with any trade adjustment, there are benefits and downsides. However, if you’re looking for a short strangle adjustment to help reduce the directional risk after a decrease in the stock price, then rolling down the short call is one option available to you.

Concept Checks

➥When selling strangles, if the share price falls towards your short put, you can adjust the position by “rolling down” the short call (buy back the old short call, sell a new call at a lower strike price).

➥By rolling down the short call, you increase the amount of option premium collected and neutralize your position delta (resulting in a lower breakeven point on the downside and less notable losses if the stock price continues to fall).

➥The downside of rolling is that you decrease the range of maximum profitability since your new call strike is closer to the short put’s strike price. Additionally, you’ll make less money (or potentially lose money) from reversals in the stock price after rolling.

Next Lesson

Additional Resources

Get a Strong Hold On Profit With Strangles – Investopedia

Chris Butler portrait

2 Conservative Option Strategies for 2022

Posted on by Chris Butler

Last updated on February 10th, 2022 , 07:28 pm

Covered Call P&L Graph

One common criticism of options trading is that the strategies are extremely risky.

While some strategies carry massive loss potential, there are two conservative option strategies that are always less risky than buying 100 shares of stock.

Option Strategies Less Risky Than Buying Stock

The two conservative option strategies that are less risky than buying 100 shares of stock are:

1. Covered Calls

2. Cash-Secured Puts

Let’s walk through each of these simple strategies.

Simple Covered Call Explanation

While we won’t get into the specific details of each strategy, we’ll cover a simple breakdown of each.

The covered call strategy consists of selling a call option against 100 shares of stock that you own.

By selling the call option, you collect “option premium,” which you keep if the stock price is below the call’s strike price at expiration.

For example, if you buy 100 shares of stock for $100 per share and sell a 110 call against it for $5.00, you collect $500 in option premium (since an option’s contract multiplier is $100, and $5.00 x $100 = $500).

What if the company goes out of business and the stock price goes to $0? Let’s compare the long stock and covered call strategies side-by-side:

covered call vs long stock

If the stock price goes to $0, the loss on a long stock position of 100 shares purchased at $100 per share would be $10,000: $100 Loss Per Share x 100 Shares = -$10,000.

However, if the stock price goes to $0, the 110 call option would also expire worthless, which means the covered call trader would have a $500 profit on the call they sold (since it was sold for $500 in premium and is now worth $0).

As a result, their net loss is $9,500: $100 Loss Per Share x 100 Shares + $500 Profit on Call = -$9,500.

In fact, at any price below $110 at the expiration date of the call option, the covered call position will outperform the long stock position by the amount of the call premium ($500 in this case).

Covered Calls vs. Long Stock

By selling a call option against 100 shares of stock, the premium collected reduces the maximum loss potential of the position.

At any price below the call’s strike price at expiration, the covered call position will outperform a long stock position by the amount of the call premium collected.

Simple Cash-Secured Put Explanation

The second conservative option strategy less risky than buying stock is the cash-secured put.

A cash-secured put refers to a short put option (a put is sold) that is fully secured by cash. For example, if you sell a put option with a strike price of $50, the maximum loss on that put option is $5,000, which means you’d need to have $5,000 in available option buying power to sell that put.

However, if you collect $2.50 ($250 in option premium) for selling that put, then you’d only need $4,750 in available option buying power to sell that put.

Let’s compare the long stock position to the cash-secured put position:

stock vs secured put

When comparing purchasing 100 shares of stock for $50 per share to selling a 50 put for $2.50, the cash-secured put position has less risk.

If the stock price goes to $0, the loss on the long stock position is $5,000: $50 Loss Per Share x 100 Shares = -$5,000.

At the same time, a put option with a strike price of $50 would be worth $50 if the stock fell to $0. However, since the put was sold for $2.50, the loss would only be $4,750: $47.50 Loss on the Put x $100 Option Contract Multiplier = -$4,750.

Cash-Secured Puts vs. Long Stock

By selling a cash-secured put option, the premium collected reduces the maximum loss potential on the position (compared to buying 100 shares of stock at that put’s strike price).

The Downsides of Covered Calls & Cash-Secured Puts

Unfortunately, it’s not all great news in regards to covered calls and cash-secured puts when compared to buying 100 shares of stock.

Unlike long stock positions, covered calls and cash-secured puts have limited profit potential, while owning shares of stock is an unlimited profit potential position (theoretically).

However, if the stock price does not increase substantially, covered calls and cash-secured put positions will outperform long stock positions, as they can profit when the stock price doesn’t increase, or even decreases slightly.

Concept Checks

Here are the essential points to remember about these two conservative option strategies:

➥ Covered calls and cash-secured puts both carry less loss potential compared to simply buying 100 shares of stock.

➥ However, both of these option strategies have limited profit potential, which means they will underperform just owning 100 shares of stock if the stock price surges.

Additional Resources

Conservative Option Strategies For 2022 and Beyond –  OptionTradingIQ

Chris Butler portrait

Why Your Percentage of Profitable Trades Means Nothing

Posted on by Chris Butler

Last updated on February 10th, 2022 , 07:29 pm

One of the appeals of options trading is that you can trade strategies with high success rates (90% of higher in some cases).

This is especially true when you sell options and take profits at small profit percentages. In fact, one of our iron condor research reports found that managing iron condors at 25% of the maximum profit potential realized a 93% success rate. Unfortunately, that high success rate was met with the lowest profit expectancy of all the management approaches.

In this post, we’ll discuss why win rates alone mean nothing.

Percentage of Profitable Trades Comparison

Let’s look at three hypothetical traders and their percentage of profitable trades over the past year:

percent profitable trades

Based on these profit percentages, which trader did the best?

It might be easy to say that Trader C performed the best, but we actually have no idea how any of the traders performed. We need two other pieces of information to find the answer.

Success Rates, Average Profits, & Average Losses

Without knowing the average profits and average losses of each trader’s positions, the percentage of profitable trades means nothing and doesn’t tell us anything about the success of each trader’s approach.

Let’s look at the same table from the previous section with the added information of average profits and losses:

comparing option traders

*(Average Profit x Win Rate) – (Average Loss x Loss Rate)

With more information, we can now see that Trader B had the best performance, despite having a “middle of the road” percentage of profitable trades.

What’s the Point?

There are two reasons for bringing up this topic:

1. Just because a strategy has a low realized success rate, it doesn’t mean it’s a bad strategy.

2. If a strategy has an extremely high success rate, it’s not necessarily a great strategy.

What truly matters is each strategy’s performance relative to what’s required to break even over time, based on average profits and average losses.

Long Premium Example

As an example, let’s say your strategy is to buy $1,000 worth of options and sell them when the options double in price (100% return). Otherwise, you’ll let the options expire worthless (100% loss).

If we assume either outcome, you’d need a 50% success rate to break even over time:

(100% Return x 50% Win Rate) – (100% Loss x 50% Loss Rate) = $0

To achieve positive trade expectancy over the long-term, one of three things needs to occur:

1. Achieve a success rate greater than 50%

2. Realize losses smaller than 100% (with the same success rate and average profits)

3. Realize profits greater than 100% (with the same success rate and average losses)

Of course, a change in one variable is likely to have an impact on the others, but the bottom line is that a strategy will only be profitable if its realized success rate exceeds the required success rate determined by the average profits and losses.

Short Premium Example

In regards to a short premium approach, let’s say you plan to take profits at 50% of the maximum profit potential or take losses when the option prices doubles (100% loss relative to the credit received).

Based on a 50% profit or 100% loss, you’d need a 66.6% success rate to break even over time (assuming all trades are sized similarly):

(66.6% Win Rate x 50% Profit) – (33.4% Loss Rate x 100% Loss) = $0

To achieve positive trade expectancy over the long-term, one of three things needs to occur:

1. Achieve a success rate greater than 66.6% (same average profits and losses)

2. Realize losses smaller than 100% (with the same success rate and average profits)

3. Realize profits greater than 50% (with the same success rate and average losses)

Same as before, a change in one variable is likely to have an impact on the others, but the bottom line is that a strategy will only be profitable if its realized success rate exceeds the required success rate determined by the average profits and losses.

The Bottom Line

The bottom line is that it’s very easy to be deceived by the percentage of profitable trades when analyzing trading strategies.

Don’t be fooled.

The success rate matters, but only relative to the required success rate as determined by the average profits and average losses over time.

Concept Checks

Here are the essential points to remember about the percentage of profitable trades:

 

1. Option strategies can have extremely low or high percentages of profitable trades, but the success rate alone means nothing

 

2. The combination of the percentage of profitable trades, average profits, and average losses can be used to determine the “best” trading strategies.

 

3. Starting from a baseline expectancy of zero, positive trade expectancy can be achieved by increasing the win rate (with the same average profits and average losses), increasing profits (with average losses and success rate being the same), or decreasing losses (with average profits and success rate being the same).

Next Lesson

Additional Resources

When and How to Take Profits on Options – Investopedia

Chris Butler portrait

Iron Condor Adjustment: Rolling Up Put Spreads

Posted on by Chris Butler

Last updated on February 10th, 2022 , 07:32 pm

Iron Condor Options Strategy

Sometimes, you’ll need to make an adjustment to your option positions when the stock price moves against you.

In this post, we’re going to discuss the iron condor adjustment strategy of rolling up the short put spreads.

Additionally, we’ll discuss the benefits of this iron condor adjustment strategy, as well as its drawbacks.

What is Rolling?

In options trading, “rolling” refers to closing an existing option position and opening a similar option position with different strike prices, in a different expiration cycle, or a combination of the two.

Today, we’ll focus on “rolling up” the short put spread in a short iron condor position, which refers to buying back your current short put spread and “rolling it up” by selling a new put spread at higher strike prices.

Rolling an Option

The process of closing an existing option and opening a similar option position at different strike prices, in a different expiration cycle, or a combination of the two.

When Do You Roll Up the Put Spread?

When trading iron condors, the most typical time to roll up the short put spread is after the stock price increases towards your short call spread.

Consider the following visual:

 

Iron Condor Adjustment: Rolling Up the Short Put Spread

As we can see, the stock price is rising quickly and approaching the short call spread portion of the trade. Since short iron condors have negative gamma, the position’s delta grows negative (the position becomes bearish) as the stock price trends towards the short call.

The most common iron condor adjustment to make in this scenario is to roll up the short put spread to higher strike prices:

 

Iron Condor Adjustment: Rolling Up the Short Put Spread

To roll up the short put spreads in an iron condor position, a trader has to buy back the old short put spread and sell a new put spread at higher strike prices.

What does this iron condor adjustment accomplish?

What Does Rolling Up the Put Spreads Accomplish?

By rolling up the short put spreads in an iron condor position, a trader accomplishes two things:

1. Collect More Option Premium

Rolling up the put spread is done for a net credit, as the new put spread is sold for more than the trader pays to buy back the old put spread. By collecting additional option premium, the maximum loss potential of the position is reduced and the maximum profit potential increases by the amount of the credit received from rolling.

However, the spreads are now closer together, which means the iron condor position has a narrower range of maximum profitability.

 

2. Neutralize Directional Exposure

By rolling up the put spreads, the iron condor’s directional exposure will shift from bearish to more neutral.

Why?

At the time of rolling up the put spreads, an iron condor will have a negative position delta, which means the trader will lose money from subsequent increases in the stock price.

When the put spreads are rolled to higher strike prices, the new put spreads will have a more positive position delta than the old spreads, which brings the iron condor’s overall delta exposure closer to zero.

Let’s cover each of these points in more depth.

#1: Collect More Option Premium

Consider a trader who is short the 130/120 put spread in their iron condor position but decides to roll up to the 145/135 put spread:

 

puts premium

How much option premium is collected by rolling up this put spread?

Premium Collected:

$2.37 Collected from 145/135 Put Spread

– $0.44 Paid for the 130/120 Put Spread

= +$1.93 ($193 Less Risk & $193 More Profit Potential)

By rolling up the short put spread, the overall premium received in this iron condor position increases by $1.93, which means the position has $1.93 less risk and $1.93 more profit potential.

However, since the new put spread is much closer to the stock price, the stock has less room to move, leading to a tighter range of maximum profitability.

#2: Neutralize Your Position Delta

After rolling up the short put spreads in an iron condor, the directional exposure becomes more neutral.

At the time of rolling, lets say the iron condor’s position delta was -25 (the trader is expected to lose $25 with a $1 increase in the stock price, and make $25 with a $1 decrease in the stock price). Here’s how the position delta would change after rolling up the put spread:​

To calculate the new iron condor delta exposure, let’s compare the old put spread’s delta to the new put spread’s delta, and add the difference to the iron condor’s current position delta of -25:

Old Put Spread Delta: +6 (+9 Short Put Position Delta – 3 Long Put Position Delta)

New Put Spread Delta: +24 (+39 Short Put Position Delta – 15 Long Put Position Delta)

Change in Put Spread Deltas: +18

New Iron Condor Delta: -25 + 18 = -7

To clarify, the put spread deltas are calculated with the following formula:

(Short Put Delta x -1 Contract x 100 Option Multiplier) – (Long Put Delta x +1 Contract x 100 Option Multiplier)

After rolling up the put spread, the iron condor’s delta exposure changes from -25 to -7, which means the iron condor is now 72% less sensitive to small changes in the stock price.

More specifically, the trader is only expected to lose $7 with a $1 increase in the stock price as opposed to a $25 loss before the iron condor adjustment.

What’s the Risk of Rolling Up the Put Spreads?

While rolling up a short put spread decreases trade risk, increases maximum profit potential, and neutralizes the iron condor’s directional exposure, there are some downsides:

1. Narrower Range of Profitability

An iron condor’s maximum profit zone lies between the short call and short put strike price. After rolling up the put spreads, the short call and short put strike prices are now much closer, which results in a much narrower range of maximum profitability.

 

2. Neutralized Directional Exposure

By neutralizing the iron condor’s directional exposure, a reversal in the stock price will yield less profits (or even losses) than before rolling up the put spreads.

As with any trade adjustment, there are benefits and downsides. The iron condor adjustment strategy of rolling up the short put spreads decreases the loss potential, increases the profit potential, but ultimately makes the trade a lower probability position since the maximum profit zone is now tighter.

Concept Checks

Here are the essential points to remember the iron condor adjustment of rolling up the short put spreads:

 

1. When trading iron condors, if the share price appreciates towards your short call spread, you can adjust the position by rolling up the put spreads to higher strike prices.

 

2. By rolling up the put spreads, you collect more option premium, which increases the profit potential and decreases the loss potential, but decreases the trade’s probability of profit since the profit zone is narrower. Additionally, the iron condor’s directional exposure becomes more neutral.

 

3. The downside of rolling up the put spreads are a decreased range of profitability, and a more neutral directional exposure. If the stock price ends up falling after the put spreads are rolled to higher strikes, the iron condor may end up losing money on the downside.

Additional Resources

Iron Condor – tastytrade

Chris Butler portrait

Short Call Management Results from 41,600 Trades

Posted on by Chris Butler

Last updated on February 10th, 2022 , 07:35 pm

Short Call Option Graph

The short call option strategy is used by bearish traders with a high risk tolerance, as the strategy has unlimited loss potential (in theory).

In this post, we’ll examine over 10 years of 16-delta short call management data from 41,600 trades in the S&P 500 ETF (SPY).

More specifically, we’ll answer the following questions:

1. Which short call management strategies were the most profitable?

2. Which short call strategies were the least profitable?

3. How did implied volatility at the time of entering the trade impact the overall profitability?

Study Methodology

Underlying: S&P 500 ETF (SPY).

Time Frame: January 2007 to May 2017 (most recent standard expiration, as of this writing).

Entry Dates: Every trading day.

Expiration Cycle: Standard expiration closest to 45 days to expiration (resulted in trades between 30-60 days to expiration).

Trade: Sell the 16-Delta call option

Number of Contracts: 1

Short Call Trade Management

For each entry, we tested 16 different management combinations:

Profit or Expiration: 25% Profit, 50% Profit, 75% Profit, OR Expiration.

Profit or -100% Loss: 25% Profit, 50% Profit, 75% Profit, OR -100% Loss.

Profit or -200% Loss: 25% Profit, 50% Profit, 75% Profit, OR -200% Loss.

Profit or -300% Loss: 25% Profit, 50% Profit, 75% Profit, OR -300% Loss.

Management Example: 50% Profit or -200% Loss

To demonstrate how we calculated the profit and loss levels, let’s run through a quick example using a 50% profit OR -200% loss target.

Entry Credit: $1.00

Profit Target: $0.50 ($1.00 Entry Credit – $1.00 x 50%)

Loss Limit: $2.00 ($1.00 Entry Credit x 200%). A $2.00 loss would occur when the call option traded $3.00 ($1.00 Entry Credit + $2.00 Loss).

Metrics We’ll Analyze

Before we get started, I want to quickly cover the primary metrics we’ll analyze:

Win Rates: The percentage of trades that were profitable.

Win Rate – Breakeven Win Rate: The difference between the success rate and what the strategy required to break even (based on average profits and losses).

Average P/L: The average profitability of each trade. The average P/L figures will be consistent with the Win Rate – Breakeven Win Rate levels.

10th Percentile P/L: The P/L that 90% of trades exceeded (a probabilistic way of analyzing the worst drawdowns).

45-Day Adjusted P/L: Not all trades were held for the same amount of time. We standardized the average P/L of each trade to a 45-day period.

Win Rates: 16-Delta Short Calls

By closing profitable short call positions early, the success rates increased. Conversely, success rates declined when closing losing short call trades early:

 

SPY Short Call Success Rates

To clarify the x-axis on these charts, I wanted to run through a quick example of how to interpret the labels. The following format is used: Profit Target / Loss Limit. If “Exp.” is present in one of those spots, then that combination had no profit target (if “Exp.” is first) or loss limit (if “Exp.” is second).

For example, “25% / Exp.” means the trades were closed for 25% of the maximum profit potential OR held to expiration (no loss management).

“Exp. / -200%” means the trades were held to expiration (no profit management) OR closed for a -200% loss.

Win Rates – Breakeven Win Rates

The Win Rate – Breakeven Win Rate metric helps us understand how each strategy performed relative to the required success rate to break even over time. For example, if a strategy’s average profits and average losses require a 75% success rate to break even, then a success rate of 80% would result in a Win Rate – Breakeven Win Rate of 5% (80% Realized Success Rate – 75% Required Breakeven Win Rate).

The metric puts more context around success rates.

Let’s take a look at the Win Rate – Breakeven Win Rate statistics for the short call trades:

 

SPY Short Call Breakeven Win Rates

As we can see, many of these short call management strategies barely cleared the required success rate that would result in breaking even over time (based on average profits and average losses).

The results make sense, as we’ve been in a strong bull market period since 2009, which means many of these short call positions were likely “blown out” as the S&P 500 moved higher in a swift fashion.

Average P/L Per Trade

Which short call management strategies were the most profitable, on average?

 

Short Call Strategy Average P/L

Based on these figures, we can see that most short call strategies in the S&P 500 have been only slightly profitable. Obviously, this can be attributed to the bull market period from 2009-2017 (the time of this writing).

Additionally, when factoring in commissions, most of these approaches may actually end up with losses, on average (depending on the commission rate).

For example, if we assume a trader’s commissions are $1 per option contract, then it costs $2 in commissions to open and close one short call contract. Based on a $2 round-trip fee, we can subtract $2 from the above Average P/L figures to get the average P/L per trade after commissions. 

10th Percentile P/L

If you recall, the 10th percentile P/L tells us the P/L level that 90% of trades exceeded. For example, if the 10th percentile P/L is -$750, then 90% of short call trades had a P/L better than a loss of $750.

Let’s take a look at these “worst-case” drawdowns for each short call management approach:

 

SPY Short Call Drawdowns

As we might expect, taking losses substantially reduced the worst-case drawdowns.

Additionally, by not taking losses and managing profits sooner (25% or 50% of the maximum profit potential), the 10th percentile P/L was worse than the larger profit-taking combinations, as the larger profits help offset the losses.

Average Time in Trade

How long was each short call position held for, on average?

 

SPY Short Call Management: Average Time in Trade

As we’d expect, incorporating some type of profit or loss management results in fewer days in each trade, on average. How does the reduced time in each trade impact the hypothetical average P/L for these short call positions over similar time periods?

To answer this question, we’ll adjust the average P/L per trade of each approach to a 45-day period:

45-Day Adjusted Average P/L = Avg. P/L Per Trade x (45 / Avg. Days in Trade)

Of course, this isn’t a perfect formula, but it does help put context around the “average profitability” of each approach based on the number of trades that can “fit” into similar periods of time.

 

SPY Short Call 45-Day Average P/L

After adjusting each short call management strategy’s average P/L per trade to a 45-day period, we find that the 25% to 50% profit targets generated the most profits, on average.

However, keep in mind that opening and closing trades more often will generate more commission costs.

What would the profitability of these strategy’s look like when taking out $2 per round-trip trade? That is, a $1 commission rate per contract multiplied by two (one trade to open the position and one to close it).

Let’s take a look:

 

SPY Short Call Management: Avg. P/L - Commissions

45-Day Avg. P/L – Commissions = 45-Day Avg. P/L – [(45 / Avg. Days in Trade) x $2]

While not a perfect estimation, the above graph should help put context around the commission impact of managing profitable trades sooner and ultimately trading more often.

These figures suggest that the 50% profit target level has historically had a good balance between the average P/L per trade, number of trades, and commissions for trading more often.

Additionally, the -100% loss limit has historically had the worst performance compared to the other short call management approaches, which suggests taking losses at -100% of the credit received has been “too soon.”

In other words, many of the short call positions that reached the -100% loss level came back and ended up as profitable trades.

Short Call Performance by VIX Level

In this final section, we’ll analyze some of the metrics from above, but we’ll evenly divide all of the trades into four buckets based on the VIX level at the time of entering the short call trades:

1. VIX Below 14

2. VIX Between 14 and 17.5

3. VIX Between 17.5 and 23.5

4. VIX Above 23.5

These VIX levels were selected based on the 25th, 50th, and 75th percentile of VIX levels at the time of all trade entries. By using these percentiles, we evenly divide all trades into four separate buckets and avoid any one bucket having substantially more or less occurrences than the rest.

Short Call Win Rates by VIX Level

At a quick glance, we can see that many of the short call management combinations had the highest success rates in the lowest and highest VIX environments:

 

SPY Short Call Management: Win Rates by VIX Level

How did these success rates compare to the required success rate to break even?

Win Rates – Breakeven Win Rates by VIX Level

As we can see, not all approaches had a win rate higher than what was required to break even (based on average profits and average losses):

 

Again, the lowest and highest VIX environments were the best-performing volatility environments for most of the short call management approaches.

Short Call Average P/L by VIX Level

Consistent with previous findings, the lowest and highest VIX environments have generated the highest average P/L per trade for most of the short call management approaches:

 

 

Interestingly, the best-performing groups included selling calls in the highest VIX environment and using a wide stop-loss (-200% or -300%) combined with high profit targets (75% or hold to expiration).

10th Percentile P/L by VIX Level

Similar to the short put management results, the largest short call losses occurred in the highest VIX entries:

 

 

When the VIX is high, it’s typically due to a recent downturn in the market. Since 2008, nearly every significant market dip (and therefore VIX spike) has been met with a strong and quick rally to the upside, which causes problems for short call positions.

45-Day Adjusted Average P/L by VIX Level

When normalizing each approach’s average P/L per trade to a 45-day period, we get the following results:

 

45-Day Average P/L = Avg. P/L Per Trade x (45 / Avg. Days in Trade)

Based on the data, closing profitable short call positions sooner (25-50% of max profit) has typically performed the best in the lowest VIX entry category, suggesting that holding longer for more profits has led to more short call positions getting “blown out” as the S&P 500 moved higher.

The short call management approaches that performed the best in the highest VIX entries included a generous stop-loss (-200% or -300%) with early profit management.

Checklist

While we’ve covered a ton of data in this post, here are the most important findings:

✓ Historically, the 16-delta short call strategy has performed best in high VIX environments when paired with a high profit target (50-100%) and a generous stop-loss (-200% or -300%)

✓ When factoring in a $1 commission per option contract, many of the short call management approaches analyzed in this study were not profitable. The ones that were probably were not worth the risk, as the profits after commissions were so small (resulting in very poor risk/reward).

✓ Lastly, the largest short call drawdowns have occurred in high VIX environments, which explains why adding a -200% or -300% stop-loss has been beneficial for high implied volatility entries.

Next Lesson

Additional Resources

Short Call Strategy Guide – OptionAlpha

Chris Butler portrait

7 Best Options Trading Tips for Beginners in 2022

Posted on by Chris Butler

Last updated on February 10th, 2022 , 07:38 pm

As an options trading beginner, there’s so much to learn and it can be overwhelming. In addition, there are common pitfalls many beginner traders experience.

In this video, we’ll give you our top 7 options trading tips for beginners, which should help you make better trading decisions (and less mistakes) when starting out.

Chris Butler portrait

Implied Volatility in Options for Beginners (Guide w/ Visuals)

Posted on by Chris Butler

Last updated on February 10th, 2022 , 07:41 pm

Implied volatility is a crucial options trading concept for beginners to understand, but it can be a daunting thing to learn because it seems very complex.

While the math behind calculating implied volatility is complex, all we need to know as options traders is this:

➜ Implied volatility quantifies option prices and expresses those option prices as one number.

Instead of looking at 100s of option prices on various stocks and wondering whether they’re “cheap” or “expensive,” we can look at implied volatility to quickly assess a stock’s option prices, and what the market thinks about that stock in terms of risk.

Understanding Implied Volatility: What Do Option Prices Represent?

Before talking about why implied volatility changes, let’s quickly discuss what option prices actually represent:

➜ Option prices are representative of the market’s anticipation of a stock’s volatility over some period of time in the future.

When trying to understand why implied volatility changes, we need to take one step back and think about why option prices change.

The #1 Driver of Option Price Changes

Perhaps the #1 general driver of option prices changes is historical/realized volatility, or how large a stock’s recent price movements have been.

To verify this statement, I plotted the relationship between 1-month historical volatility of the S&P 500 and the VIX Index, which measures 1-month option prices on the S&P 500:

 

VIX Index vs. Historical Volatility (SPX)

The horizontal axis (x-axis) represents the 1-month historical volatility of the S&P 500 Index (SPX) on each trading day since the VIX Index began.

The vertical axis (y-axis) represents the VIX Index closing value on each of the days the historical volatility was recorded. When plotted against each other, there is a clear relationship between historical volatility and implied volatility (option prices):

➜ When the market is experiencing more volatility (higher 1-month historical volatility readings), the 1-month option prices on the S&P 500 tend to be higher as well (higher 1-month option prices = higher VIX Index).

➜ When the market is less volatile (left side of chart), option prices are cheaper.

Let’s look at some real market examples and validate this idea even further.

Market Volatility vs. Option Prices

In the chart below, we’ve placed a box around a very low volatility period. As we can see, the market was grinding higher during this period and did not experience any large decreases:

 

Software Used: tastyworks

The very end of the boxed region in the chart above is November 1st, 2018.

On that day, we looked at the 30-day straddle price on SPY.

Here are the relevant statistics:

SPY Closing Price: $257.49

Expiration/Options Analyzed: 30 Days to Expiration

257.50 Straddle Price: $4.59 (1.78% of SPY’s Closing Price)

VIX Index (30-Day Implied Volatility): 10.2%

After a period of low volatility, the 30-day straddle on SPY was trading for 1.8% of SPY’s closing price, and the VIX Index was at 10.20.

Let’s look at the same exact metrics in a high volatility market.

The chart below features an extremely high volatility period. The end of the boxed region is December 20th, 2018:

Software Used: tastyworks

Here are the relevant statistics from December 20th, 2018:

SPY Closing Price: $247.17

Expiration/Options Analyzed: 29 Days to Expiration

247 Straddle Price: $13.86 (5.6% of SPY’s Closing Price)

VIX Index (30-Day Implied Volatility): 28.50%

As we can see, the at-the-money straddle price was significantly higher after this particular high volatility period, as the 29-day straddle was trading for 5.6% of SPY’s closing price. As a result, the VIX Index was at 28.50.

Comparison: Option Prices in High vs. Low Volatility Markets

Let’s compare each volatility environment and the respective option prices side-by-side:

 

low volatility vs high volatility

Clearly, when markets are calm, option prices are cheaper because there’s not nearly as much perceived risk compared to when the market is very volatile. 

If you can imagine a transition from Scenario #1 (low volatility) to Scenario #2 (high volatility), then you will understand why option prices change.

Just like insurance policies, an increase in health risks means the insurance company will require higher premium payments from the individual being insured. The same concept holds true in regards to option prices.

Implied Volatility and Earnings

There’s one special case where implied volatility “increases” but option prices stay the same or decrease.

When a stock has an earnings announcement approaching, the stock’s option prices in the expiration cycle immediately after earnings will remain inflated, as sometimes stocks make big movements after earnings reports.

Because of this, the option prices exposed to that potentially large movement remain inflated, even as their expiration dates rapidly approach. Let’s look at an example from AAPL’s earnings in November of 2018:

 

aapl expiration options

In the table above, we can see that the at-the-money straddle price in the earnings expiration cycle actually lost some value as the expiration date approached.

However, even with the slight decrease in option prices, implied volatility increased from 50% to 103%!

The reason for this is implied volatility is a function of not just option prices, but how much value the options have relative to their time to expiration.

In the event where option prices remain constant as time passes, implied volatility will increase. That’s because with less time to expiration and the same option prices, the market is expecting higher levels of volatility when adjusted for time.

In other words, a ±$10 expected move over one trading day will result in a significantly higher implied volatility reading compared to a ±$10 expected move over 60 trading days.

Next Lesson

Additional Resources

What Is Historical Volatility? – Fidelity Investments

Chris Butler portrait

Option Vega Explained (Guide w/ Examples & Visuals)

Posted on by Chris Butler

Last updated on May 3rd, 2022 , 08:48 am

Option Vega

Option Vega Definition: In options trading, the Greek “Vega” (Greek letter v) measures an option’s sensitivity to implied volatility. Vega tells us how much the option premium of a derivative will increase by when volatility increases by 1%.

What Is Vega In Options Trading?

Before we get into what an option’s vega is, let’s review the biggest risks every options trader must be aware of:

1) Changes in the price of the stock (directional risk – delta)

2) Changes in the directional risk of a position (gamma risk)

3) The passing of time (referred to as time decay or theta decay)

4) Changes in implied volatility of the underlying asset (volatility or vega risk)

5) Changes in interest rates (Rho)

Vega is the option Greek that relates to the fourth risk, which is volatility or vega risk. More specifically, vega estimates the change in an option’s price relative to changes in implied volatility.

Read! Historical Volatility vs Implied Volatility

Vega is always presented as a positive number because as option prices increase, implied volatility increases (all else equal). Conversely, as option prices decrease, implied volatility decreases. Let’s go through some examples of how vega is applied.

Care to watch the video instead? Check it out below!

Basic Option Vega Example

Vega is expressed as an option’s expected price changes relative each 1% (absolute) changes in implied volatility.

In the following table, work your way from left to right, and notice how an option’s price is expected to change relative to movements in implied volatility:

option vega table

As you can see, an option vega of 0.25 represents a $0.25 increase in the option’s price per 1% increase in implied volatility, and vice versa. With a 3% decrease in implied volatility, the option’s value is expected to be $0.75 lower.

In order to estimate an option’s expected price relative to a 1% increase in implied volatility, simply add the option’s vega to its price. For 1% decreases in implied volatility, an option’s price can be estimated by subtracting vega from its price.

Nice! You’ve learned the basics of an option’s vega! We’ve just got one more section to go. Next, you’ll learn about the options with the most vega risk.

Which Options Have the Most Vega?

In this section, we’re going to analyze various call options and put options to determine which ones have the most exposure to changes in implied volatility.

To do this, we’ll run a series of tests. First, we’re going to look at option vega values at each strike price. Then, we’ll analyze the vega of options with shorter and longer amounts of time until the expiration date. Let’s do it!

Option Vega vs. Strike Price

To illustrate which options have the most exposure to vega, we picked a random day in 2016 and graphed the vega of each out-of-the-money (OTM) call and put. We used the expiration cycle with nearly 50 days to expiration. Let’s take a look at the results!

 

Option Vega vs. Strike Price

As illustrated here, option contracts closest to the underlying stock price (at-the-money or “ATM”) have the highest vega values. In this particular example, the at-the-money options are expected to be worth $0.28 more with implied volatility 1% higher, and vice versa. On the other hand, an out-of-the-money put with a strike price of 170 is expected to increase by only $0.07 relative to each 1% increase in implied volatility, and vice versa.

Another thing this graph tells us is that an option’s vega is related to the amount of extrinsic value it has because at-the-money options have the most extrinsic value, and out-of-the-money options have the least amount of extrinsic value.

Read! Determining the Price Of An Option – Intrinsic Value vs Extrinsic Value in Options

Alright, we’ve checked off the first analytical test. Now, we’re going to build on this by analyzing the vega of each option over multiple expiration cycles.

Vega vs. Time to Expiration

Similar to before, we’re going to graph the vega of each out-of-the-money option, but this time we’ll do it for three expiration cycles. The cycles we chose were 15, 71, and 225 days to expiration, respectively. Let’s check it out!

 

Option Vega vs. Days to Expiration

As we can see here, options with more time until expiration have larger vega values. This means that longer-term options are expected to have more volatile price changes relative to implied volatility changes. Again, this makes sense because longer-term options have more extrinsic value.

To understand why options with more extrinsic value have higher vega values, consider the following hypothetical scenario:

Now, if these option prices both went to $0, implied volatility would be 0%. In order to reach $0, Option B has to lose $0.75 while Option A only has to lose $0.25. Therefore, Option B has a larger vega value.

Vega Calculation Using Black Scholes

According to columbia.edu, the below pricing model formula satisfies Vega:

vega formula calculation

Note! Trading options come with great risks. To better understand the risks of standardized options, please read this article from the OCC.

Option Vega FAQs

Vega tells us how much the price of an option will change in response to a 1% change in the implied volatility of the underlying. Option prices rise with implied volatility. 

In options trading, a negative vega implies a net short position. This applies for both short single options and short spreads. 

Options that are trading at-the-money are most sensitive to changes in implied volatility. Therefore, at-the-money option have a higher vega than in-the-money and out-of-the-money options. 

Back To Greeks Home ➥

Next Lesson

Additional Resources

Vega Definition – Investopedia

Options Vega – The Greeks – CME Group

Chris Butler portrait