February 2022

What is Position Delta? | Options Trading Concept Guide

Posted on February 3, 2022April 29, 2022 by Chris Butler

Last updated on April 29th, 2022 , 06:02 am

The delta of an option expresses that option’s expected price change relative to movements in the stock price. For example, a +0.50 delta call option is expected to gain $0.50 in value when the stock price increases by $1. Conversely, that same option is expected to lose $0.50 when the stock price falls by $1.

However, it’s important to know how that option price change translates to profits and losses for your position, especially when trading complex positions with more than one option. Position delta takes things a step further and estimates the profits or losses on an entire option position relative to $1 changes in the stock price.

Care to watch the video instead? Check it out below!

Option Delta Chain

In the above options chain, the call price should increase from $10 to $10.75 with a $1 increase in the underlying stock price, and decrease from $10 to $9.25 with a $1 decrease in the stock price.

But how does this translate to profits or losses for a trader with -2 contracts (short two contracts)?

Since this trader is short the call options, they profit from price decreases. More specifically, a decrease from $10 to $9.25 represents a $0.75 profit per option contract.

Recall that an option represents 100 shares of stock, so we need to multiply the change in the option price by 100 to solve for the actual return in dollars:

($10 sale price – $9.25 current price) x 100 = +$75

Lastly, the trader in this example is short two contracts, so the $75 profit becomes a $150 profit when multiplying by two contracts.

Working through this example, we learn that the trader is expected to profit by $150 when the stock price decreases by $1. Therefore, the trader’s position delta in this example is -150, as a $1 increase in the stock price should lead to $150 in losses, and a $1 decrease in the stock price should result in a profit of $150.

So, while the option’s delta is +0.75, it doesn’t indicate the expected profits or losses for a trader who is short two contracts. Analyzing the overall delta of the position solves this problem by converting an option’s delta into the expected profits or losses for a specific position when the stock price changes.

Alright, so you know the basic idea behind position delta. Next, we’ll discuss the formula you can use to calculate the delta for any option position.

Need a brokerage account?

We trade with tastyworks because of their trader-friendly commissions ($10 options commission cap and close trades for free) and easy-to-use platform.

Sign up with tastyworks and earn up to $500 for funding your first account ($200 for funding $2,000 -OR- $500 for funding $10,000).

Offer valid through 2/28/2022

Calculating Position Delta

To calculate the position delta for a standard equity option position, the following formula can be used:

The following table demonstrates this formula applied to various simple option positions:

It’s important to note that when selling options or shares of stock short, the number of contracts traded is a negative number. Using negative numbers to indicate short positions is conventional in the trading industry.

Here are the main points to take away from this table:

➜ When short contracts (negative number of contracts), the position delta will have the opposite sign of the option’s delta.

➜ A share of stock has a delta of +1.00, which results in positive position delta when buying shares and negative delta when shorting shares.

➜ A positive position delta represents a position that profits from stock price increases and loses money from stock price decreases. For example, the +630 delta position is expected to profit by $630 with a $1 increase in the share price and lose $630 after a $1 decrease in the share price.

➜ A negative position delta represents a position that profits from stock price decreases and loses money from stock price increases. For example, the -83,700 delta position is expected to lose $83,700 with a $1 increase in the share price and profit by $83,700 with a $1 drop in the share price.

At this point, we’ve discussed position delta and how to calculate it for simple positions. In the next section, we’ll calculate the delta of more complex positions.

Position Delta of Complex Positions

Calculating the delta of a more complex option position is simple. All you have to do is sum up the position delta for each option in the strategy. As an example, let’s calculate the overall delta for a hypothetical long call spread:

In this example, the five long calls generate a delta of +375 while the five short calls generate a delta of -125. Adding these two deltas together leaves us with a net position delta of +250.

Next, we’ll calculate the overall delta for a hypothetical short iron condor option strategy, which is a strategy that includes four different options:

In this example, the long puts and calls generate -30 and +30 deltas respectively, resulting in zero delta exposure. However, a 0.35 call and a -0.25 put are sold, resulting in a slightly bearish bias. The net position delta of this iron condor is -30, which indicates an expected $30 profit when the stock price falls by $1 and a $30 loss when the stock rises by $1.

Real Trade Examples

In the following example, we’ll take a look at the delta and profit/loss of an option position that recently traded in the market. We’ll visualize the performance of the position and examine the accuracy of position delta.

SPY Short Puts

In this example, we’ll examine the position delta and P/L of a short put option position in SPY. The position was initiated in February of 2016 and the options expired in March of 2016.

To make things easier for you, we’ve shaded two regions that demonstrate the accuracy of position delta. The following table compares the expected vs. actual P/L based on the position delta in each region.

As we can see here, the actual P/L was fairly close to the expected P/L that was projected by the position delta. The differences in each case can be attributed to the fact that position delta changes when the stock price changes. Additionally, other factors like theta decay and changes in implied volatility also contribute to P/L.

What is Delta Neutral?

In options trading, delta neutral is a strategy used to balance out both positive and negative delta. This is accomplished through both buying and selling shares of the underlying stock.

The goal of this strategy (used by both market makers and professional option traders) is to offset the risk that comes with holding an inventory of call options and put options.

In a true delta neutral portfolio, the net delta of an entire position will be zero.

Position Delta FAQs

What is the delta of a portfolio?

In options trading, the delta of a portfolio tells us how sensitive a security is to changes in the underlying price. Assuming all other variables are constant, delta tells us the amount an option price is expected to move based on a $1 future change in the underlying stock price.

What is a good Delta in options?

There is no such things as a “good” delta in options. Traders who want to take on high risk generally have larger net deltas while traders wanting low risk, or a reduction in risk, have deltas approaching zero.

Next Lesson

Additional Resources

Position Delta and ways to manage it – CNBC

Top 8 Benefits of Trading Options

Posted on February 3, 2022February 14, 2022 by Chris Butler

Last updated on February 14th, 2022 , 09:41 am

So, you’ve read a couple of articles about options trading before, or maybe you have some friends that trade them, but you haven’t yet taken the plunge. What’s all the hype?

In this post, projectfinance is going to share our list of the top eight benefits of trading options.

TAKEAWAYS

When compared to stock, options require less capital.
Options are usually leveraged at a ratio of 100:1, meaning one contract represents 100 shares of stock.
Options allow investors to profit from any market direction.
Using metrics such as the “Greeks”, options trading lets investors choose their trade’s probability of success.
Options trading allows investors to reduce their overall risk in various positions.
You can combine stocks and options together in strategies such as the “covered call”.
Unlike stock, options are generally short-term trades. This forces traders to be in sync with the undulations of the market.

#1: Lower Capital Requirement

Investing in shares of stock is expensive, especially for young investors who don’t have a lot to work with. For example, consider the capital requirement for the stock investments in the following table:

Stock Price	Shares Purchased	Capital Required
$100	50	$5,000
$250	75	$18,750
$500	100	$50,000

*Cash Account

As you can see, buying shares of stock requires significant sums of money, especially for young investors who are just getting started. Sure, you could buy a few shares here and there, but the stock prices will need to appreciate substantially to experience any decent returns, and buying a low number of shares is extremely inefficient in terms of commissions.

With options, you can implement strategies that require a few hundred dollars to enter. Here are two hypothetical option positions you could enter that don’t require significant sums of money to implement:

Options Trade	Capital Required	Profit Potential	Max Loss
Sell $2-Wide Iron Condor For $1.00	$100	$100	$100
Sell $3-Wide Put Spread for $1.00	$200	$100	$200

As you can see, some options strategies can be implemented with a very low capital requirement. However, it’s important to note that you should always consider the potential loss on your portfolio if a position turns out to be a loser.

#2: Options Give You The Ability to Use Leverage

The second reason investors should learn to trade options is that options give you the ability to use leverage. While leverage is a weapon of mass destruction for the unprepared and naïve, leverage can be powerful when implemented properly (and with a plan).

Let’s take a look at how options can provide leverage:

Stock	Stock Price	100 Share Cost	At-The-Money 1-Year Option Cost
AAPL	$117	$11,700	$1,200
TSLA	$203	$20,300	$3,300
GOOGL	$800	$80,000	$7,500

As we can see here, buying 100 shares of any of these stocks costs between $10,000 and $80,000. However, an investor could purchase an at-the-money call option and control 100 shares of each stock for a year at a fraction of the cost. In each case, using a call option to gain exposure to 100 shares of stock can be achieved at 10% of the cost of buying shares.

Let’s look at the return potential on the AAPL call option from the previous table based on various stock prices in one year:

Stock Price in One Year	Return on 100 Shares (Bought at $117/Share)	Return on 115 Call (Purchased for $1,200)
$50	-$6,700 (-57%)	-$1,200 (-100%)
$117	$0 (0%)	-$1,000 (-83%)
$135	+$1,800 (+15%)	+$800 (+67%)

As you can see, buying a call option instead of stock provides immense return potential when the stock price increases. Additionally, a call option has limited loss potential when the stock price decreases.

However, buying a call option will be unprofitable if the stock price doesn’t increase by a certain amount, and maybe you don’t want to implement a strategy that loses money when the stock price doesn’t change.

Let’s look at the profit and loss potential on a 60-days until expiration options strategy that requires $750 to implement. Again, we’ll use AAPL options for the example:

Stock Price in 60 Days	Return on 100 Shares (Bought at $117/Share)	Return on 115/105 Put Spread (Sold for $250; $750 Maximum Loss)
$100	-$1,700 (-15%)	-$750 (-100%)
$117	$0 (0%)	+250 (+33%)
$125	+800 (+7%)	+$250 (+33%)
$130	+$1,300 (+11%)	+$250 (+33%)

With this particular options strategy, a return of 33% can be achieved in 60 days without any change in the stock price. Additionally, when the stock price collapses, the losses are less significant than owning 100 shares of stock. What’s the catch? Well, the profit potential of the options strategy is limited, whereas owning shares of stock has unlimited profit potential.

In summary, options give investors the ability to implement strategies with a low cost of entry. Additionally, strategies can be customized to profit from certain scenarios, which brings us to the next reason every investor should learn options trading.

#3: Customize Your Strategy

As you saw in the previous examples, options provide investors with ways to customize strategies based on their investment theories regarding a particular stock. Here are a few ways options trading can be custom tailored:

Have a trade time frame as short-term as a few hours or as long-term as two years.
Profit when the stock price increases, remains in a specified range, decreases, or even moves against your position slightly.
Profit primarily from the passing of time, or changes in the level of fear in the marketplace.
Generate income each month on shares of stock you own while waiting to sell those shares at a higher price (with the covered call strategy).
Generate a stream of income each month while waiting to buy shares of stock at a lower price (with the put-selling strategy).

As an example, check out the performance of this strangle options strategy that profits as long as the stock price remains in a specified range:

The chart above illustrates just one example of how options can be used to customize your strategy. Aside from customizing your specific strategy, options allow you to choose the estimated probability of making money on a particular trade.

#4: You Can Choose Your Probabilities

When purchasing shares of stock, the share price must increase for you to make any money. On a short-term basis, the probability of a stock rising or falling from its current price is estimated at 50%. This means the probability of making money from buying or shorting stock is approximately 50%.

With options, the estimated probability of making money can be below or above 50%. More specifically, options traders can choose the estimated probability of making money on a trade based on the risk and reward relationship of their proposed strategy.

For example, traders who primarily sell options typically have more loss potential than reward potential, and therefore have a probability of profit that’s greater than 50%. On the other hand, traders who buy options typically have more profit potential than loss potential, and therefore have a probability of profit that’s lower than 50%.

So, if you’re a trader who prefers to have limited risk and high reward potential, your strategy will have a low probability of profit. On the other hand, if you’re ok with having more loss potential than reward, your strategy will have a high probability of profit. Either way, you can choose which side of the equation you want to be on, and even balance high probability trades with some low probability trades.

#5: Options Can Be Used to Reduce Risk

When most people hear about options, they typically hear about how incredibly risky they are. Frankly, these comments are warranted, as many individuals abuse options and implement them with highly risky approaches that are doomed to fail eventually.

However, options can be (and often are) used to reduce the risk of an existing stock or option position. Let’s look at an example of how options can be used to eliminate the loss potential of a long stock position below a certain price:

As we can see here, a put option is used to reduce the losses of a stock position below a certain price. Without the put option, the loss is $5,000 at the worst point. However, the stock position that is protected by a put option only experiences a loss of $2,368 at the worst point.

Here’s an example of how call options can be used to reduce the risk of a long stock position:

In this example, it’s clear that the covered call position (selling a call against 100 shares of stock) performed better than the stock position the entire time. More importantly, the losses on the covered call position were always less than the losses on the stock position by itself.

The last two examples demonstrate how simple implementations of options can reduce the risk of existing positions.

#6: You Can Combine Options With Stock Investing

Reason #6 for why investors should learn to trade options is that you don’t have to choose options trading or stock investing. You can do both!

Options are a perfect complement to stock investments. After all, options are derivatives of stocks, which just means their prices are derived from the stock that they’re traded against.

As a stock investor who understands options, you will:

Know how to use options to create a stream of monthly income on the shares you already own.
Understand how to reduce the risk of, or lock in profits of a profitable stock position.
Be able to calculate the estimated probabilities of specific stock price changes over any period of time.
Have the ability to gauge how the market perceives the riskiness of a particular stock by looking at the stock’s option prices.

There are many benefits of knowing options as a stock investor. Always remember that you don’t have to abandon buy-and-hold stock investing for options trading, you can do both. Why limit yourself?

#7: You'll Be More In Tune With the Economy

As a stock investor, it’s much easier to buy some shares and forget about the market for months at a time because stock investments are typically long-term.

As an options trader, there’s a good chance most of your trades will be short-term in nature (typically less than 60-90 days). As a result, you’ll be actively placing, adjusting, and closing trades. With more exposure to the markets through your positions, you’ll be more in tune with events related to the stocks that you’re trading options on, as well as macroeconomic events

With more exposure to the markets, there’s a higher probability that you stumble upon other attractive trading or investing opportunities.

#8: Trading Options is FUN

Investing in the markets is exciting in general, but there’s nothing quite like the freedom or flexibility that comes with the ways you can apply options trading to your portfolio. It doesn’t matter whether you trade options for risk reduction, aggressive speculation, or steady monthly income, trading options is fun, plain and simple. Everybody likes a little fun, right?

Final Word

As opposed to stock trading, options offer investors both leverage and flexibility. However, these benefits do indeed come with added risks.

Options trading requires diligence. The set-it-and-forget-it approach does not work here. If you’d like to jump-start your education on learning about calls and puts, please check out our comprehensive article below, Options Trading for Beginners.

Next Lesson

Additional Resources

Benefits & Risks of Options Trading | Nasdaq

The Cost of Trading Volatility: VXX In Depth

Posted on February 3, 2022March 18, 2022 by Chris Butler

Last updated on March 18th, 2022 , 06:13 am

When trading volatility products, it’s imperative that you’re aware of the costs associated with the positions you enter. More specifically, being aware of the current VIX term structure (VIX futures curve) as it relates to historical levels can help you make more informed volatility trading decisions.

My First Volatility Mishap

In May of 2014, I had just purchased my first VIX futures contract. The reason for the trade was that implied volatility was low and the market had been grinding steadily higher without any breathers to the downside, and I anticipated a reversion in volatility. At the time, I had overlooked the fact that VIX futures converge towards the VIX Index as time passes, and when volatility is low, VIX futures typically trade at a premium to the VIX. The result? Steady losses on long VIX futures contracts (and other long volatility strategies/products) when market implied volatility remains low.

If you look at the graph below, you’ll notice that the front-month VIX futures are consistently above the VIX Index when the VIX is at suppressed levels (a structure referred to as “contango”):

Data compiled from Yahoo! Finance and CBOE VIX Futures Data.

When the VIX term structure is in contango, long volatility traders pay the price, and must correctly time the inevitable volatility increase to avoid the costs of contango. If the timing is off, the losses from contango lead to an increase in the cost basis of long volatility trades, which means a more significant increase in volatility is needed to break even or profit.

NOTE! In March, 2022, Barclay’s suspended sales and issuance of VXX. Read about other volatility finds in our article: “VXX Alternatives“.

Historical VIX Term Structure Levels

So, how often is the VIX futures curve in contango and backwardation? Here are the historical spreads between the front-month VIX Future and the VIX Index since 2008:

As we can see, the difference between the front-month VIX future and the VIX Index is typically positive, indicating that the VIX Index is at a discount to the front-month VIX future. Let’s take a look at some metrics that describe the spread’s history since 2008:

Interestingly, the median spread between the front-month VIX future and the VIX Index has been +0.80. When purchasing a VIX future at an $0.80 premium to the VIX Index, the potential loss from holding the contract when volatility doesn’t expand is $800 ($0.80 Loss Per Contract x $1,000 Contract Multiplier). The higher the spread, the higher the potential costs of buying volatility.

VIX Term Structure and Daily VXX Performance By Year

VIX futures are not alone in terms of the costs of trading volatility. A popular volatility ETN under the ticker symbol VXX tracks the performance of the two nearest-term VIX futures contracts. The result? Poor performance when the VIX futures curve is in contango (which, as we know, is the case most of the time).

Let’s take a look at the median premium of the front-month VIX future (M1) to the VIX Index, the median relationship between the second-month VIX futures contract (M2) and the front-month VIX futures contract, and the median daily VXX changes:

Just to clarify, the M2 / M1 relationship is important because VXX tracks an index that “rolls” the front-month futures (M1) to the second-month futures (M2) on a daily basis. When the M2 contract is at a more significant premium to the M1 contract, it’s an indication that near-term contango is steep and that VXX faces larger losses if the VIX remains low.

Implications for Trading Volatility

As we can see from this data, the contango has been very steep in 2017 (and since the U.S. election) relative to previous years. What are the implications of this? Well, steep contango will lead to more significant losses when trading volatility to the long side if the VIX remains suppressed, requiring more accurate timing of increases in market volatility.

So, while market implied volatility is currently at an extreme, understand that the costs associated with trading long volatility positions are high relative to historical norms. Does this suggest that shorting volatility is the better play? While short volatility strategies will perform considerably well if the VIX structure remains in its current state, there’s always the potential for a significant spike in volatility, especially with the VIX below 12. So, the risk/reward for shorting volatility at these levels won’t make sense for most traders.

At the very least, it’s interesting to see how significant the current volatility risk premium is relative to historical levels, and it should help volatility traders make more informed trading decisions.

Next Lesson

Additional Resources

CBOE Volatility Index: Live Data

Implied Volatility and Calendar Spreads

Posted on February 3, 2022February 14, 2022 by Chris Butler

Last updated on February 14th, 2022 , 09:43 am

The long calendar spread is an options strategy that consists of selling a near-term option, while simultaneously purchasing a longer-term option at the same strike price. Calendar spreads can be constructed with calls or puts.

Since both options use the same strike price, they’ll both always have the same amount of intrinsic value, which means a calendar spread attempts to take advantage of the extrinsic values of each option.

A Long Calendar Spread’s Profit Drivers

When analyzing the position Greeks of a long calendar spread, we find that the position has positive theta and positive vega. As a result, a calendar spread can profit in two ways:

#1: The passage of time while the underlying price remains close to the strike price of the calendar. As time passes, the short option should lose more value than the long option, generating profits for a long calendar spread trader.

#2: An increase in implied volatility. The longer-term option has a higher vega value than the near-term option, which means the long option should gain more value than the short option when implied volatility increases.

Consider the following example trade:

As we can see from the example calendar spread, the position has positive theta and positive vega. The positive theta value of +$0.11 means a trader who owns this calendar spread should theoretically make $11 ($0.11 x 100) in profit with each day that passes, all else being equal. The positive vega value of +$1.22 indicates that the long calendar spread trader should profit by $122 ($1.22 x 100) if each option’s implied volatility increases by 1%. There’s just one problem: short-term and long-term implied volatilities (option prices) do not typically change at the same rate.

What if the short put’s implied volatility increases by 2% and the long put’s implied volatility increase by 1%? In this scenario, the long calendar trader would actually be expected to lose money from the volatility increase: (-$2.74 Short Option Vega x 2 Point IV Increase) + ($3.96 Long Option Vega x 1 Point IV Increase) = -$5.48 + $3.96 = -$1.52. So, despite the long calendar spread’s positive vega of +$1.22, a trader who owned the calendar spread is expected to lose $152 if the short option’s IV increases by 2% while the long option’s IV only increases by 1%.

Near-Term vs. Long-Term Option Price Sensitivity

To illustrate the difference between short-term and long-term option price (implied volatility) sensitivities, we can look at implied volatility indices that track SPX option prices with various amounts of time until expiration. Here are the three volatility indices we’ll examine:

By tracking the values of each index over time (especially through a period in which SPX implied volatility increases), we can learn how option prices with various amounts of time until expiration change relative to each other.

Let’s take a look at how each of these volatility indices changed through the market correction in August of 2015:

When the S&P 500 Index (SPX) began to collapse in late August, we can see that each of the volatility indices increased, indicating an increase in SPX option prices across the board. However, we can see that the nearest-term SPX options (as quantified by VXST) experienced the largest increase in implied volatility, while the longer-term SPX options (as quantified by VXMT) did not experience the same volatility increase.

So, despite the fact that long calendar spreads trade with positive vega, they can actually lose money from an increase in implied volatility. However, since significant increases in implied volatility tend to occur when the market crashes, a long calendar spread will likely be a losing position anyways, as the market will be moving away from the calendar’s strike price.

Trade Example #1: Directional Long Calendar Through a Stock Market Correction

Let’s look at some real long calendar examples to demonstrate the concepts discussed above.

The first example we’ll look at is a bearish long calendar spread in August of 2015. The short option is the 1,970 put expiring in September 2015, and the long option is the 1,970 put expiring in October 2015. On August 20th, the September 1,970 put had a vega of +1.99, while the October 1,970 put had a vega of +2.94. Let’s see what happened to the calendar spread after the market fell to the calendar’s strike price:

Based on the +1.99 vega of the September 1,970 put, a 3.2% increase in implied volatility should result in a $6.37 increase in the short put’s price (+1.99 x +3.2 = +$6.37). With a vega of +2.94, a 2.2% increase in the October 1,970 put’s implied volatility should result in a $6.47 increase in the long put’s price (+2.94 x +2.2 = +$6.47). Since a long calendar spread trader is short the near-term option and owns the longer-term option, the changes in implied volatility only account for $0.10 of the profits on the spread ($6.37 loss on the short option + $6.47 profit on the long option = +$0.10 profit).

The additional $1.05 in profits can be explained by the calendar spread’s bearish directional bias, as the delta of the spread was -0.04 on August 20th.

So, the moral of the story is that even though the stock price fell to the calendar strike and implied volatility increased, the price of the long calendar spread only increased by 8.7% (with most of the gains coming from the spread’s directional bias). This is because the near-term implied volatility increased more than the long-term implied volatility, resulting in less of a volatility impact than the Greeks initially indicated.

Trade Example #2: Long Calendar Spread Profits from an IV Decrease

As we discussed earlier, near-term option prices (implied volatility) are much more sensitive than longer-term option prices (implied volatility). Knowing this, can long calendar spreads actually profit when volatility decreases, despite having positive vega?

To answer this question, let’s look at a long calendar spread that’s entered in a period of high implied volatility. More specifically, where the near-term IV is at a premium to the longer-term IVs (a condition referred to as backwardation). In this example, we’ll look at a long 1,860 SPX call calendar spread between January 20th and January 22nd of 2016. On January 20th, 2016, the February 1,860 call had a vega of +2.12, and the March 1,860 call had a vega of +2.95. Let’s look at the trade metrics from entry until two days after:

Based on the +2.12 vega of the February 1,860 call, a 2.3% decrease in implied volatility should result in a $4.88 decrease in the short call’s price (2.12 x -2.3 = -$4.88). With a vega of +2.95, a 1.3% decrease in the March 1,860 call’s implied volatility should result in a $3.84 decrease in the long call’s price (+2.95 x -1.3 = -$3.84). As a result, the net profit from the volatility changes alone is +$1.04 per calendar spread ($4.88 profit on the short call’s price decrease – $3.84 loss on the long call’s price decrease = +$1.04).

So, despite having a vega of +0.83, the long calendar spread actually made money from the decrease in implied volatility. The volatility-related profits can be explained by the fact that the short option’s implied volatility decreased more than the long option’s implied volatility.

Now, since SPX rallied away from the calendar’s strike price of $1,860, the trade began to incur directional losses that offset the profits from the volatility decrease, which is why the overall gain was only $0.55. Either way, this example serves as a nice demonstration as to how a long calendar spread can actually profit from an implied volatility decrease.

What’s the Point?

So, what’s the point of all of this? First, and perhaps the biggest thing I want you to take away from this post is that calendar spreads may not be good long volatility trades, even though they trade with positive vega. Additionally, calendar spreads lose their ability to profit when the stock price rises or falls significantly, with the latter being the cause for most significant implied volatility increases. In my opinion, long calendar spreads are really designed to take advantage of low realized volatility (small movements in the underlying), in which case profits occur from the front-month option decaying at a faster rate than the back-month option.

Second, even though long calendar spreads trade with positive vega, they may actually be suitable for entries in extremely high implied volatility. Why? Because you’re selling inflated near-term implied volatility and buying a cheaper longer-term implied volatility. If the market trades sideways for one or two days and volatility falls, the near-term implied volatility should fall faster than the longer-term implied volatility, translating to quick profits for a long calendar spread trader. However, if the only goal is to trade the near-term vs. longer-term IVs, a “cleaner” trade can be constructed with VIX futures calendar spreads (for larger accounts), or synthetics in VIX options (to trade with smaller size).

Summary of Main Concepts

To quickly summarize what this post has covered, here are the key points to remember:

A long calendar spread has positive theta and positive vega, which means the position profits from the passage of time (as long as the stock price is near the strike price), and an increase in implied volatility (if both options experience a similar increase in implied volatility, or the long option experiences a larger increase in implied volatility).
Though a long calendar spread has positive vega, losses can actually occur from IV increases, as near-term IV typically rises faster than longer-term IV.
In the same vein, long calendar spreads can actually profit from falling implied volatility, as near-term IV typically falls faster than longer-term IV. This type of setup is most common after a severe market correction, in which case the near-term IVs will trade at a significant premium to the longer-term IVs (a condition called backwardation).

Next Lesson

Additional Resources

What is a Calendar Spread Option? | tastytrade

Selling Option Strangles: When to Hold and Fold

Posted on February 3, 2022February 16, 2022 by Chris Butler

Last updated on February 16th, 2022 , 01:49 pm

When starting out in trading, it’s entirely natural to focus on the profit side of the equation. However, losses in trading are inevitable, and keeping losses manageable is the key to long-term profitability.

In this post, we’re going to compare two trading approaches and see which one has performed the best. Since we’re an options trading website, we’re going to focus on a short premium (selling options) approach and compare two separate trade management plans when selling strangles:

1. Only exit trades if a pre-defined profit target is reached. Otherwise, hold to expiration.

2. Only close trades if a pre-defined loss limit is reached. Otherwise, hold to expiration.

We’ll also look at the combination of these two strategies.

Let’s briefly discuss the methodology we’ll use for this analysis.

Study Methodology

Underlying: S&P 500 ETF (SPY)

1. All positions entered on the first trading day of each month from 2007 to present.

2. Select standard options expiration cycle in the following month (43-52 days to expiration).

3. Sell a strangle with a 16-delta call and 16-delta put.

Now, for each strangle position we tested two separate management approaches:

1. Close profitable strangles for 50% of the maximum profit potential. For example, if a strangle was sold for $1.50, the trade was only closed if the strangle’s price fell to $0.75 (a 50% profit). If the profit target is never hit, hold the position until expiration and let any losses run.

2. Close unprofitable strangles for -100% of the maximum profit potential. For example, if a strangle is sold for $2.00, the position was only closed if the strangle’s price reached $4.00 (a loss equal to 100% of the maximum $2.00 profit potential). If the stop-loss is never hit, hold the position until expiration and let any profits run.

We also added the combination of these two strategies for comparison.

Study Results: Taking Profits vs. Taking Losses

After running the study, we rounded up the cumulative profit/loss of each management approach. Here’s what we found:

SPY Short Strangles: Taking Profits vs. Taking Losses

As we can see here, only taking losses at the pre-determined loss limit resulted in an end profit 76% higher than the approach in which only profits were taken.

Let’s look at some of the profitability metrics related to each management approach:

While the loss-taking approach had a 17% lower success rate, the strategy significantly outperformed in the profit expectancy and worst drawdown categories.

By taking losses, you get stopped out of some losing trades that end up being profitable, which leads to a lower success rate. However, when the losing trades don’t come back, taking the loss results in keeping a larger portion of the profits from previous trades.

By taking profits and losses, the combined management strategy got the best of all worlds: high success rate, small drawdowns, and similar profitability to the trades that were closed for a profit or held to expiration.

When implementing any short premium approach, profits are typically small and frequent. However, the losses are infrequent and substantial. The key to any options trading approach (especially a short premium approach that utilizes undefined-risk strategies) is minimizing drawdowns and keeping most of the small, frequent profits.

In this post, we covered a very basic management approach applied to short strangles. The small study we presented is only one test using one specific strategy, but the notion of avoiding large losses is the most important takeaway.

Summary of Main Concepts

Here are the main concepts to take away from this post:

Many traders focus on the profit side of the equation, though focusing on losses can be more beneficial.
When selling 16-delta strangles in the S&P 500 over the past 10 years, closing only losing trades performed significantly better than only closing profitable trades.
Though the loss-taking approach to selling strangles had an 18% lower success rate, the strategy made up for it by keeping the losses small (the minimum drawdown was 80% lower than simply taking profits and holding losing trades).
When implementing an option-selling strategy with significant loss potential, profits will typically be small and frequent, but the infrequent losses can be substantial. Avoiding catastrophic drawdowns when selling options is the key to long-term profitability.

Next Lesson

Additional Resources

Get a Strong Hold On Profit With Strangles – Investopedia

How Options Volatility Products Work: VXST, VIX, VXV, VXMT

Posted on February 3, 2022March 15, 2022 by Chris Butler

Last updated on March 15th, 2022 , 09:42 am

The S&P 500 is the most-watched U.S. equity index among traders and investors around the world, as it is perhaps the best representation of the U.S. stock market. Not surprisingly, the options on the S&P 500 Index (SPX) are extremely active, as market participants can use SPX options to trade predictions related to the overall market, or hedge a portfolio of stocks efficiently.

Since the prices of options can serve as an indication of marketplace fear or complacency, traders and investors around the world like to pay attention to the levels of SPX option prices, as well as any changes in those option prices as the value of the S&P 500 and economic conditions change.

To quantify SPX option prices, we can refer to the CBOE’s many volatility index products.

What Are VXST, VIX, VXV, and VXMT?

There are four primary CBOE volatility index products related to the S&P 500 Index options. Each of the following indices quantify the prices (implied volatility) of SPX options with varying lengths of time until expiration:

Each of these volatility indices can be used to gauge demand for S&P 500 options over different time frames.

Short-Term vs. Long Term Implied Volatility: Calm Market Periods

So, what is the “normal” relationship between these volatility indices? When markets are calm, near-term implied volatility typically trades at a discount to longer-term implied volatility (VXST < VIX < VXV < VXMT).

The following chart demonstrates the typical near-term and long-term implied volatility relationship when markets are calm:

As we can see, the CBOE Short-Term Volatility Index (VXST) is at a significant discount to the CBOE Mid-Term Volatility Index (VXMT). In other words, demand for short-term options is much less significant than the demand for long-term options.

What explains this relationship? When implied volatility is low, it’s usually because the market’s realized movements on a day-to-day basis are small. With minuscule market movements, there’s less demand for protection in the form of SPX options over all time frames because smaller daily market movements translate to more certainty and less fear.

However, there’s less certainty over longer periods of time, which explains why longer-term option prices tend to trade with higher levels of implied volatility.

Short-Term vs. Long Term Implied Volatility: Fearful Market Periods

During extremely fearful market periods this relationship inverts, as demand for short-term protection increases much faster than the demand for long-term protection. We can visualize this by looking at VXST, VIX, VXV, and VXMT into the market correction of August 2015:

SPX Implied Volatilities: VXST, VIX, VXV, VXMT

As we can see, VXST, VIX, VXV, and VXMT all shifted higher, but near-term implied volatility increased the most. Here’s a snapshot of these volatility indices on August 24th, 2015:

In this particular snapshot, near-term SPX option prices are pumped up to an implied volatility that is significantly higher than the longer-term SPX option prices.

During highly volatile market periods, there’s more fear and less certainty, which translates to more demand for protection. When fear becomes the dominant force in the marketplace, short-term options tend to trade at higher implied volatilities than longer-term options because there’s less certainty in the near-term, but also an expectation that the fear will eventually subside (as indicated by VXV and VXMT trading at a discount to VXST and VIX).

During a period of high market volatility, the expectation of less volatility in the future is similar to that of a human’s emotions. When somebody gets angry, it’s typically only a short burst. In time, the person cools down and returns to a “normal” state. It’s the same thing with market volatility.

By using VXST, VIX, VXV, and VXMT, we can keep an eye on the “temperature” of the market, and gain more context around the market’s demand for short-term and long-term options.

Summary of Main Concepts

To quickly summarize what this post has covered, here are the key points to remember:

Option prices can help us take the “temperature” of the market. To quantify the prices of options on the S&P 500, we can keep an eye on VXST, VIX, VXV, and VXMT.
VXST is the CBOE Short-Term Volatility Index, which tracks the implied volatility of S&P 500 Index options with 9 days to expiration.
The VIX is the CBOE Volatility Index, which tracks the implied volatility of S&P 500 Index options with 30 days to expiration.
VXV is the CBOE 3-Month Volatility Index, which tracks the implied volatility of S&P 500 Index options with 93 days to expiration.
VXMT is the CBOE Mid-Term Volatility Index, which tracks the implied volatility of S&P 500 Index options with 6-9 Months During calm market periods (small daily market movements), short-term options (quantified by VXST) typically trade at a lower implied volatility than longer-term options (quantified by VXMT). This relationship indicates more certainty in the near-term and less certainty over the long-term to expiration.
During volatile market periods (large daily market movements), short-term options (quantified by VXST) typically trade at a higher implied volatility than longer-term options (quantified by VXMT). This relationship indicates more fear and less certainty in the near-term, and an expectation that market volatility (and fear) will subside to a more “normal” level over the long-term.

Next Lesson

Additional Resources

CBOE Volatility Index: Live Data

Historical Volatility Explained: Is it Useful to Options Traders?

Posted on February 3, 2022February 10, 2022 by Chris Butler

Last updated on February 10th, 2022 , 12:28 pm

Most options traders tend to focus solely on implied volatility, which makes sense, as implied volatility is a forward-looking indicator based on the prices of a stock’s options. By analyzing implied volatility, options traders can determine the market’s expected price range for a stock in the future, as well as assess the current levels of option prices relative to historical “norms” for each particular underlying.

What is Historical Volatility?

Some traders like to also look at historical volatility, which is the annualized standard deviation of a stock’s past returns (usually daily returns). For example, if the standard deviation of a stock’s returns over the past 20 trading days (one month) is 2%, then the annualized 20-day historical volatility would be 31.7%:

2% Standard Deviation of Past 20 Daily Returns x SQRT(252 Trading Days Per Year)

= 31.7% 20-Day Historical Volatility

Refer to this article to learn more about calculating and interpreting historical volatility.

Implied Volatility vs. Historical Volatility

Historical volatility can help traders understand a stock’s past price movements, which can then be compared to the expected price movements of the stock in the future (via implied volatility or the stock’s option prices).

For example, consider a scenario where a stock’s options are trading at a 20% implied volatility, but the stock’s 20-day historical volatility is only 10%. In this case, traders might view the stock’s options as a good sale since the options are implying a 20% annualized movement while the stock’s past returns are much less volatile.

On the other hand, if a stock’s options are trading at a 15% implied volatility, but the stock’s 20-day historical volatility is 25%, then traders might look to buy options because the option prices are lower than they should be (based on the volatility of the stock’s past movements).

Here’s a quick graph that shows the S&P 500 Index’s historical volatilities relative to the VIX Index:

Source: Yahoo! Finance

The above visual helps explain why the VIX has been trading at such a low level: the S&P 500’s realized movements have been minuscule. So, even with the VIX between 10-12.5, SPX options were still “overpriced” relative to the realized movements in SPX.

So, is historical volatility worth our attention as options traders, or should we exclusively look at implied volatility? For our first attempt at answering this question, we performed a simple test.

Study Methodology: Selling Straddles Based on the IV/HV Relationship

While there’s a great deal of research that can be conducted on this topic, today we’ll start with a basic test using short straddles on the S&P 500 ETF (SPY).

Here’s the methodology we used to test the validity of using historical volatility in the decision to enter a trade:

1. From 2007 to present, we compared S&P 500’s one-month implied volatility (the VIX Index) to the S&P 500’s one-month (20-day) historical volatility (HV).

2. On each trading day, we “sold” the at-the-money straddle in the standard expiration cycle with 25-35 days to expiration. If a standard expiration cycle did not meet that time frame, we skipped the date. This was done to keep an approximate 30-day trade time frame (since we are comparing one-month IV and HV).

3. Lastly, we divided all of the occurrences into four buckets based on the IV/HV relationship on the entry date:

VIX at a 50% Premium to the 20-Day HV
VIX at a 25-50% Premium to the 20-Day HV
VIX at a 0-25% Premium to the 20-Day HV
VIX Below the 20-Day HV

Each bucket had a similar number of trades.

Results: Entries Based on the IV/HV Relationship

Let’s take a look at various metrics related to the short straddle trades entered in each environment:

Based on this data, we can see that the trades entered when 20-day HV was below the VIX had noticeably better performance than the trades that were entered when 20-day HV was above the VIX. Most notably, the median expiration profit/loss was between 17-34% for the trades entered when HV was below IV. The trades that were entered when HV was above IV had a median expiration profit/loss of 10%.

Additionally, the trades entered when HV was above IV had slightly lower success rates and frequency of profitable trading days, though these differences were much smaller than the expiration profit/loss figures.

Despite selling options with the highest average VIX levels (the most expensive options of the four buckets), selling 30-day SPY straddles when SPY’s 20-day historical volatility exceeded the VIX resulted in decreased performance relative to the trades that were entered when SPY’s 20-day historical volatility was below the VIX.

While this simple test certainly doesn’t put the nail in the coffin on the topic of using historical volatility for trade entries, it does indicate that the idea has legs.

Summary of Main Concepts

To quickly summarize what this post has covered, here are the key points to remember:

Implied volatility is a forward-looking indication of a stock’s expected price movements based on the prices of the stock’s options. Historical volatility is a backward-looking indicator that quantifies the annualized standard deviation of a stock’s past price changes.
Some traders prefer to only look at implied volatility, while some like to analyze implied volatility and historical volatility together.
Based on our preliminary analysis, 30-day SPY short straddles entered when the S&P 500’s implied volatility exceeded its 20-day (one-month) historical volatility outperformed trades in which the 20-day historical volatility exceeded the current implied volatility.
The findings suggest that premium sellers may benefit from selling options when implied volatility exceeds the 20-day historical volatility.
In a similar vein, premium buyers may benefit from buying options when the 20-day historical volatility exceeds the current implied volatility.

Next Lesson

Additional Resources

What Is Historical Volatility? – Fidelity Investments

Choosing Option Strike Prices for Debit Spreads

Posted on February 3, 2022February 10, 2022 by Chris Butler

Last updated on February 10th, 2022 , 12:30 pm

One of the difficult parts of learning how to trade options is getting comfortable with strike price selection for various strategies. At first, the amount of strike prices available can be overwhelming, but in time the process of selecting strike prices becomes natural.

In today’s guide, we’ll discuss our process for selecting strike prices when we trade debit spreads. If you’d like a refresher on the debit spreads we’ll be focusing on, we’ve put together the following strategy guides:

1. Call Debit Spread (also referred to as a “long call spread” or “bull call spread”)

2. Put Debit Spread (also referred to as a “long put spread” or “bear put spread”)

There are two primary ways in which we structure our debit spread trades, which we’ll break down in this guide.

Selecting Strike Prices for Stock-Replication Debit Spreads

The first way we’ll structure debit spreads is with stock replication in mind. This means we’ll aim to structure our debit spread to have a similar breakeven price as the current stock price. We use this type of spread when we want long or short stock exposure but with less loss potential and the opportunity for a high return on capital. To structure this type of debit spread, we purchase an in-the-money option and sell an out-of-the-money option. The long and short options should both be similarly distanced from the stock price. Here’s an example:

In this case, buying the 134/138 call debit spread with the IWM ETF at $136.28 gives us a breakeven price of $136.25 (Long Call Strike of $134 + Spread Entry Price of $2.25 = $136.25). So, if the stock price remains right at its current level, the call spread will break even, similar to holding the actual stock. Furthermore, the maximum loss potential is $225 ($2.25 x 100) per spread, and the maximum profit potential is $175 [($4 Spread Width – $2.25 Purchase Price) x 100] per spread. For this spread, the margin requirement would be the loss potential of $225, which means the potential return on capital is 78%.

Here’s a visualization of a call debit spread that is structured similarly to the call spread in the table above:

As we can see, the spread’s breakeven price is right near the initial stock price, and the spread doesn’t lose much value when the stock price doesn’t move too much (during the period between 82 to 45 days to expiration). This is because the in-the-money option consists of mostly intrinsic value, which does not decay. Additionally, the short call’s price decay offsets most of the decay of the long option’s extrinsic value.

In short, structuring a debit spread with an in-the-money long option and an out-of-the-money short option minimizes the exposure to losses from time decay. Additionally, the spread’s probability of profit is approximately 50% because the breakeven price is right near the stock price at the time of trade entry.

Selecting Strike Prices for Lower Risk, Higher Return Debit Spreads

The second type of debit spread setup we use is to structure a strategy with asymmetric return potential, but with less risk and a higher probability of profit than simply buying a call or put. The spread is structured by purchasing an at-the-money option and selling an out-of-the-money option against it. This setup will result in less loss potential, more profit potential, and a lower probability of success.

But how do you choose the short strike in the spread? That depends on your outlook for the stock. A logical placement for the short strike is your “best-case” scenario for the stock’s movement. In other words, the furthest you think the stock will move by the spread’s expiration.

For example, the following spread uses a short strike of 130, which means the trader who buys this debit spread believes $130 is a realistic price target over the time frame of the trade:

As you’ll notice, the breakeven price of this put spread is $134.16, which is $1.91 below the current stock price. Since we need IWM to fall in order to break even at the time of this spread’s expiration date, the spread has a lower probability of profit.

In regards to potential profits and losses, this spread’s price is $1.84, which means the maximum loss potential is $184 ($1.84 x 100) per spread. Since the spread is $6 wide, its maximum potential value is $6, which means the maximum profit potential is $416 ([$6-Wide Spread – $1.84 Purchase Price] x 100) per spread.

Since the margin requirement of this spread is the maximum loss potential of $184, the potential return on capital for this trade is 226% ($416 Profit Potential / $184 Spread Purchase Price). So, by altering our strikes to structure a more directional trade with better return potential and less loss potential, the potential return on capital increased in comparison to the previous spread. However, with more return potential and less loss potential, the probability of making money on the spread decreases.

Here’s an example of a put debit spread structured similarly to the put spread from above:

Selecting Strike Prices Put Debit Spread

As we can see, the stock price hovers right around the long put’s strike price of $226, and therefore the spread as a whole begins to lose value over time. This is because the long put’s value is all extrinsic, which decays over time.

While the short option also consists of all extrinsic value, the debit spread loses value over time because the long option has more extrinsic value than the short option, and therefore loses more from time decay.

In short, buying a debit spread with an at-the-money long option and an out-of-the-money short option results in less risk and more profit potential than a debit spread with an in-the-money long option. However, the more favorable risk/reward results in a lower probability of success because the stock price has to move by a certain amount in a specific direction. If the stock doesn’t make that favorable move, the spread will lose money from time decay.

Adjusting Strike Prices to Add or Reduce Risk

Once you’ve determined the general structure of the debit spread you want to trade, the final step is to adjust the strikes to tweak the risk of the trade:

1. To increase the risk and reward of a debit spread, widen out the distance between strike prices.

2. To reduce the risk and reward of a debit spread, narrow the distance between the strike prices.

Consider the following positions:

The two spreads are very similar in regards to their breakeven prices and risk/reward ratios. However, they differ in size. The wider spread has more loss potential and more profit potential than the narrower spread.

For smaller accounts targeting lower-risk trades, debit spreads with less distance between the strike prices can be traded. For larger accounts targeting higher-risk trades or a more efficient use of commissions, wider spreads can be traded. Adjust the strike prices until the spread meets your particular risk preference.

Keep an Eye on the Short Option’s Price

The last topic we’ll discuss is the price of the short option in the debit spread. The point of trading debit spreads is to gain bullish or bearish exposure with less risk and a higher probability of profit than simply buying a call or put. The downside of trading a debit spread as opposed to a call or put is that the debit spread has limited profit potential.

Because of this, you’ll want to make sure the option you’re selling against your long option brings in enough premium to justify capping your profit potential.

Consider the following trade:

In this particular position, the short 145 call is only bringing in $0.40 in premium, which means the profit potential of the long 130 call is being limited by an option that’s only reducing the cost of the long call by $0.40 (a 5% in reduction the long 130 call’s price).

In this scenario, it may not make sense to sell the 145 call at all. If you’re going to limit the profit potential of your long call by selling a call against it, then make sure the short option’s premium justifies limiting the profit potential.

It will depend on the structure of the debit spread, but as a general guideline, the short option should bring in premium equal to or greater than 20% of the long option’s price.

Summary of Main Concepts

To quickly summarize what this post has covered, here are the key points to remember:

To structure a debit spread with a breakeven price near the current stock price, purchase an in-the-money option and sell an out-of-the-money option that are similarly distanced from the stock price. This type of spread will be similar to buying or shorting shares of stock, but with lower profit/loss potential and a higher potential return on capital.
To structure a debit spread with low-risk and high return potential, buy an at-the-money option and sell an out-of-the-money option against it. This type of setup will have lower loss potential, higher profit potential, but more exposure to losses from time decay and a lower probability of success.
To increase the risk and reward of a debit spread, widen out the distance between the strike prices.
To reduce the risk of a debit spread, decrease the width of the distance between the strike prices.
Always be sure to check the premium of the short option in a debit spread. The short option in a debit spread is meant to reduce the cost of the long option. If the short option is too cheap, it doesn’t make sense to sell the option, as the premium collected doesn’t justify capping the profit potential.
As a general guideline, the short option should bring in premium equal to or greater than 20% of the long option’s price.

Next Lesson

Additional Resources

What Is the Strike Price of an Option? thestreet.com

Selling SPY Option Straddles | In-Depth Study

Posted on February 2, 2022April 5, 2022 by Chris Butler

Last updated on April 5th, 2022 , 06:27 am

Selling straddles (a short straddle) consists of selling a call and put option at the same strike price and in the same expiration cycle. Typically, the at-the-money strike price is used because the short call and short put deltas will offset (at least initially), resulting in a directionally-neutral position.

Selling at-the-money straddles can be profitable when the stock price remains within a specific range around the straddle’s strike price, and/or when implied volatility falls.

Likelihood of Max Profit & Large Losses

Since the maximum profit potential of a short straddle position only occurs if the stock price is right at the straddle’s strike price at expiration, realizing max profit on a short straddle is very unlikely.

On the other hand, large losses on short straddle positions occur when the stock price moves substantially outside the expected move, or implied volatility surges (typically combined with a notable market selloff). Both of which do not happen often.

The Study

With the previous points in mind, we wanted to know how often short straddle positions have historically reached specific profit and loss levels in relation to the initial entry price. The historical probabilities of profit and loss levels can help set realistic management expectations.

Here’s the methodology we followed for our short straddle profit and loss study:

1. Analyzed straddle positions in the S&P 500 ETF (SPY) from January 2007 to present.

2. On every trading day with a standard expiration cycle between 25-35 days to expiration (DTE), we “sold” an at-the-money straddle. We chose 25-35 DTE to target an approximate one-month straddle.

3. For each straddle position, we recorded the maximum profit and worst loss as a percentage of the entry price.

For example, if a straddle was sold for $1, a maximum profit percentage of 50% means the lowest price the straddle reached was $0.50 ($1 Entry Price x 50%). On the other hand, a loss percentage of 70% means the highest price the straddle reached was $1.70 ($1 Entry Price + $1 Entry Price x 70%).

Results: Short Straddle P/L Frequencies

Let’s first look at the overall profit/loss frequencies for all of the 823 short straddle trades with 25-35 days to expiration:

SPY Short Straddle Profit & Loss Frequencies

As we can see from the above results, less than 50% of historical short straddle positions with 25-35 DTE reached profits greater than 50%, which can be explained by the fact that the stock price must remain in a tight range around the stock price to reach the higher profit levels.

Perhaps the most important finding is that at almost all of the profit/loss levels, a higher percentage of short straddles reached profits than losses of equal magnitude (i.e. 84% of trades reached a 20% profit but only 51% reached a 20% loss). This demonstrates that the market typically stays within the expected move, which leads to a high frequency of profits for short premium strategies.

How does implied volatility impact the results? Let’s look at the P/L frequencies for trades entered in the lower 25th percentile and upper 25th percentile of VIX levels over the test period.

P/L Frequencies: Low & High IV Environments

To quantify “low” or “high” implied volatility entries, we calculated the lower 25th percentile and upper 25th percentile of VIX levels on the days in which trades were entered:

P/L Frequencies: VIX Below 14

Let’s start by looking at the profit/loss frequencies for the short straddles entered when the VIX was below 14 (the bottom 25% of VIX levels on the days of trade entries):

SPY Short Straddles: Profit/Loss Frequency w/ VIX Below 14

Interestingly, more of the short straddles reached the higher profit levels (50%+) when entered with a VIX below 14, but more of the straddles also reached the larger loss levels. An explanation for this is that when the VIX is at a lower extreme, it’s usually accompanied by abysmally low historical volatility, which just means the market’s daily movements are small. The smaller market movements translate to steadily decaying short straddles that don’t take much heat (assuming the market isn’t surging).

However, in the event that the market initiates an outsized move from a low VIX environment, the loss on a short straddle as a percentage of the entry credit can be high. For example, if a 200 straddle is sold for $5 in a low VIX environment and SPY is trading for $190 or $210 at the straddle’s expiration date, the loss on the straddle would be 100% of the credit received (since the straddle would be worth $10 but it was sold for $5).

However, if the 200 straddle was sold for $8 in a higher VIX environment, the loss on the straddle would only be 25% if SPY was trading for $190 or $210 at the time of the straddle’s expiration date (since the straddle would be worth $10 but it was sold for $8).

The above examples help explain why the loss levels were reached at a higher frequency when the straddles were sold in a low VIX environment.

P/L Frequencies: VIX Above 23

Here are the profit/loss frequencies of the SPY short straddles that were entered when the VIX was above 23 (the top 25% of VIX readings on the days of trade entries):

SPY Short Straddles: Profit/Loss Frequency w/ VIX Above 23

Of the three entry buckets (all entries, low VIX entries, and high VIX entries), the high VIX entries had by far the best results. As we can see, high VIX short straddle entries in SPY had substantially higher frequencies of profits at each level and lower frequencies of losses at each level.

When the VIX is high, it is an indication that one-month S&P 500 options are more expensive, which means straddles are more expensive. As a result, larger market movements are required for the short straddles to reach the higher loss levels. The opposite is true for low VIX short straddle entries (as discussed in the previous section).

Additionally, since high VIX environments have typically been short-lived since 2008, the short straddles entered in high VIX environments have benefitted from the volatility contractions as market volatility subsided.

Final Comparison

We’ll end with a side-by-side comparison of the most frequent profit/loss levels (10-50%) in each of the three entry filters (all entries, VIX below 14, VIX above 23). Let’s start with the profit frequencies:

And the loss frequencies:

As we can see, the low and high VIX environments showed improvements in the realized probabilities of short straddle positions that hit specific profit and loss levels. More specifically, the profit levels were reached at a higher rate and the loss levels were reached at a lower rate in both the low VIX and high VIX entries.

The high VIX entries had the highest percentage of trades that reached most profit levels, and the lowest percentage of trades that hit the same loss levels. As a result, selling straddles in high VIX environments over the past 10 years has rewarded those willing to take on the risk, as each spike in volatility was short-lived and premium sellers reaped the benefits of selling options with elevated prices.

Closing Thoughts

On a final note, keep in mind that we’ve primarily been in a low volatility environment since the financial collapse of 2008. During sustained periods of high implied volatility (and historical volatility), the results of the above tests may differ significantly.

When trading short straddles, be sure to have pre-defined profit and loss targets, and keep trade size small. As a lower-risk alternative, short iron butterflies can be traded to gain exposures similar to a short straddle but with limited loss potential. Hopefully, the above study can help guide trade management levels in different volatility environments for these types of trades.

Summary of Main Concepts

To quickly summarize what this post has covered, here are the key points to remember:

Since the stock price needs to be very close to a short straddle’s strike price at expiration, realizing maximum profit potential is a low probability event. In the same vein, the stock price needs to shift substantially for a short straddle to book a significant loss in relation to the entry price.
Historically speaking, less than 50% of one-month short straddles in SPY have reached the 50% profit level.
Regardless of the entry environment, one-month short straddles in SPY have reached each profit level more often than loss levels of the same magnitude (e.g. short straddles have reached 20% profits more often than they’ve reached 20% losses). This can be explained by the fact that the market trades within the expected move most of the time, resulting in frequent profits for premium sellers.
Short straddles entered with the VIX above 23 (the top 25% of VIX readings over all of the trade entry dates) realized the highest percentage of trades that reached each profit level and the lowest percentage of trades that reached each loss level. The strong performance can be attributed to short-lived VIX spikes, which have translated to profits from volatility contractions and time decay simultaneously.

Curious how the strangle compares to the straddle? Check out our article here, Straddles vs Strangles.

Next Lesson

Additional Resources

What is a Straddle? Definition of Straddle, Straddle Meaning – economictimes.indiatimes.com

Iron Condor Management Results from 71,417 Trades

Posted on February 2, 2022April 25, 2022 by Chris Butler

Last updated on April 25th, 2022 , 07:11 am

Selling iron condors is an extremely popular options strategy among income traders. In this guide, you’re going to see historical profitability results for the short iron condor strategy on the S&P 500 ETF.

Watch the video below for the key takeaways, or continue reading for all of the results.

Iron Condor Setups

While there are unlimited variations of the iron condor that we could test, we had to choose only a few, as the amount of data would be overwhelming for more than one or two variations. Here are the two iron condor variations that we’ll examine in this round of analysis:

1) 16-Delta Short Options & 5-Delta Long Options

2) 30-Delta Short Options & 16-Delta Long Options

By placing the short call and put at the 16-delta level, we sell “one standard deviation” options. By definition, one standard deviation encompasses 68% of outcomes around the average (the “average” being the current stock price, in this case).

As it relates to our short iron condors, selling one standard deviation options means we have an approximate 68% probability of the iron condor expiring worthless, which occurs when the stock price is in-between the short strikes at expiration.

In the case of the second iron condor setup, 30-delta options are at the half standard deviation level. Since the short strikes are much closer to the stock price, there’s a much lower probability that the iron condors expire worthless, since there’s a lower probability that the stock price is in-between the short strikes at expiration. Based on the call and put having an approximate 30% probability of expiring ITM, the estimated probability of these iron condors expiring worthless is about 40% (100% – 30% Call Prob. ITM – 30% Put Prob. ITM).

Iron Condor Management Combinations

For the sake of completeness, we’ll be testing 16 different iron condor management combinations for each short iron condor in this study:

1) Expiration (No Management)

2) Expiration OR -100% Loss

3) Expiration OR -200% Loss

4) Expiration OR -300% Loss

5) 25% Profit OR Expiration

6) 50% Profit OR Expiration

7) 75% Profit OR Expiration

8) 25% Profit OR -100% Loss

9) 50% Profit OR -100% Loss

10) 75% Profit OR -100% Loss

11) 25% Profit OR -200% Loss

12) 50% Profit OR -200% Loss

13) 75% Profit OR -200% Loss

14) 25% Profit OR -300% Loss

15) 50% Profit OR -300% Loss

16) 75% Profit OR -300% Loss

Each management level is based on the entry credit for the iron condors. For example, if an iron condor is sold for $1.00, a -200% loss would be when the loss is 200% of the entry credit, or $2.00. A loss of $2.00 would occur when the iron condor is worth $3.00 ($1.00 Entry + $2.00 Loss = $3.00).

Regarding profits, if an iron condor is sold for $1.00, a 75% profit occurs when the profit on the trade is $0.75, which occurs when the iron condor is worth $0.25 ($1.00 Entry – $0.75 Profit = $0.25).

By testing all of these iron condor management combinations, we can isolate performance changes by taking profits, taking losses, and the combination of the two.

Study Methodology: 16-Delta Iron Condors

Underlying: S&P 500 ETF (SPY)

Time Frame: January 2007 to March 2017

Entry Dates: Every trading day

Expiration Cycle: Standard expiration closest to 45 days to expiration (resulting in trades between 30-60 days to expiration).

Iron Condor #1: Sell the 16-delta call and 16-delta put. Buy the 5-delta call and 5-delta put.

Iron Condor #2: Sell the 30-delta call and 30-delta put. Buy the 16-delta call and 16-delta put.

Number of Contracts: 1

One important thing to mention is that these iron condors have more risk on the put side than the call side, as the downside volatility skew results in a wider put spread relative to the call spread.

For example, as I write this, the iron condor in SPY with the above setup is the 225/212 put spread ($13 wide) and the 243/247 call spread ($4 wide). In the future, we can test equal-width spreads.

Iron Condor Management Combinations

For the sake of completeness, we’ll be testing 16 different iron condor management combinations for each short iron condor in this study:

1) Expiration (No Management)

2) Expiration OR -100% Loss

3) Expiration OR -200% Loss

4) Expiration OR -300% Loss

5) 25% Profit OR Expiration

6) 50% Profit OR Expiration

7) 75% Profit OR Expiration

8) 25% Profit OR -100% Loss

9) 50% Profit OR -100% Loss

10) 75% Profit OR -100% Loss

11) 25% Profit OR -200% Loss

12) 50% Profit OR -200% Loss

13) 75% Profit OR -200% Loss

14) 25% Profit OR -300% Loss

15) 50% Profit OR -300% Loss

16) 75% Profit OR -300% Loss

By testing all of these iron condor management combinations, we can isolate performance changes by taking profits, taking losses, and the combination of the two.

Study Methodology: 16-Delta Iron Condors

Underlying: S&P 500 ETF (SPY)

Time Frame: January 2007 to March 2017

Entry Dates: Every trading day

Expiration Cycle: Standard expiration closest to 45 days to expiration (resulting in trades between 30-60 days to expiration).

Iron Condor #1: Sell the 16-delta call and 16-delta put. Buy the 5-delta call and 5-delta put.

Iron Condor #2: Sell the 30-delta call and 30-delta put. Buy the 16-delta call and 16-delta put.

Number of Contracts: 1

One important thing to mention is that these iron condors have more risk on the put side than the call side, as the downside volatility skew results in a wider put spread relative to the call spread.

For example, as I write this, the iron condor in SPY with the above setup is the 225/212 put spread ($13 wide) and the 243/247 call spread ($4 wide). In the future, we can test equal-width spreads.

Results: 16-Delta / 5-Delta Iron Condors

With 16 different management combinations, we’ve got a total of 40,868 iron condors included in the following results.

Let’s start with win rates.

Win Rates: 16-Delta / 5-Delta Iron Condors

Here are the percentages of profitable trades in each iron condor management approach:

Iron Condor Management: Percentage of Profitable Trades

As we would expect, the approaches with the highest success rates were the earlier profit-taking combinations. Additionally, the loss-taking approaches saw decreases in the rate of success, as more trades were closed for losses (some of which expired profitable).

However, win rates do not tell us the whole story. We can get a better idea of the most profitable approaches by comparing the realized success rate to the required breakeven success rate (based on the average profits and average losses for each approach).

Win Rates – Breakeven Rates

Here were the differences between the realized success rates and required breakeven success rates for each approach:

Iron Condor Management: Win Rates - Required Breakeven Win Rates

As we can see, even though the 25% profit approaches had the highest success rates, they outperformed their required success rates by the lowest margin compared to any other approach.

For example, in the 25% / Expiration management approach, if the success rate was 3.1% lower, the strategy would break even over time (based on the historical average profits and average losses). The higher the success rate is relative to the required breakeven success rate, the more positive the trade’s P/L expectancy will be.

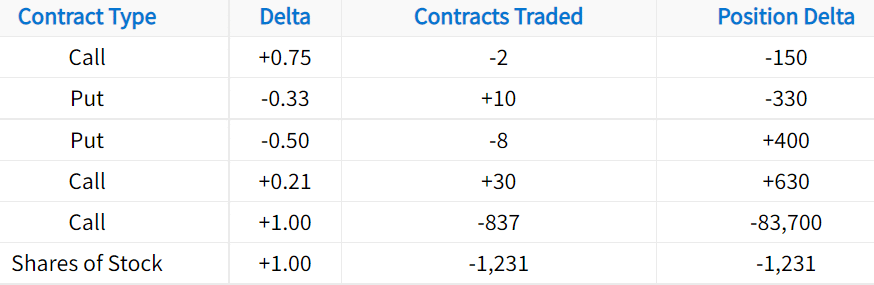
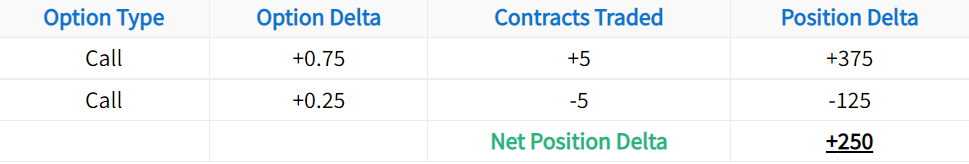
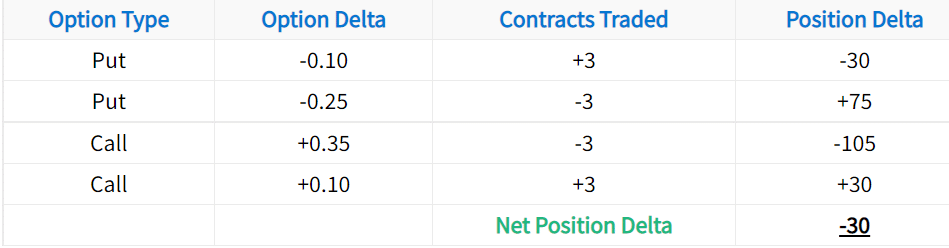
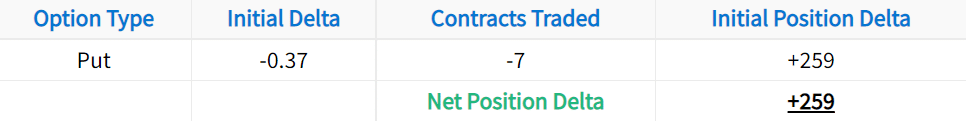
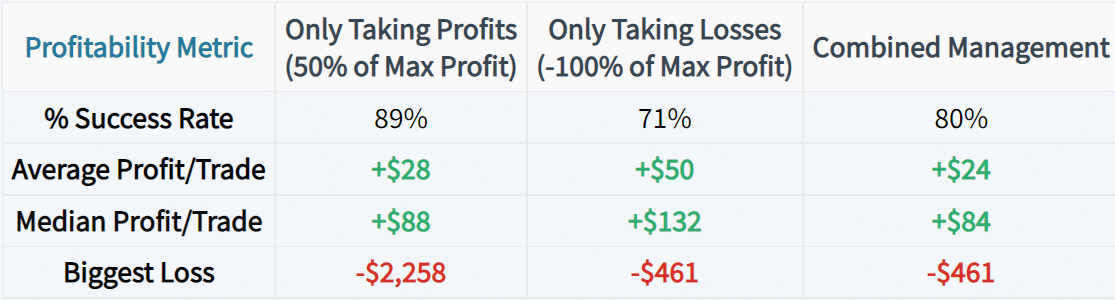
Average P/L Per Trade

Let’s take a look at the average profit/loss per trade in each iron condor management approach:

Iron Condor Management: Average Profit/Loss

The lower profit percentage approaches saw a decrease in the average P/L per trade, which is what we’d expect when taking smaller profits while also allowing the same magnitude of drawdowns.

90th Percentile Losses

To compare the outlier drawdowns of each approach, we’ll examine the 90th percentile losses for each iron condor management combination. The 90th percentile loss tells us the loss level in which 90% of losing trades did not reach. In other words, only 10% of losses were worse than the 90th percentile loss.

As expected, the tighter stop-loss approaches had the shallowest drawdowns of any approach. In regards to the profit or expiration approaches, the 25% management had the worst 90th percentile drawdown, which indicates of the few trades that were losers, the losses were typically substantial. Losses were consistent in the loss-taking approaches.

Average Time in Trades

Though some iron condor management approaches have substantially higher average profits per trade, not all trades are held for the same amount of time. So, we need to account for the fact that more trades can be squeezed into each 45-day period when closed earlier for profits or losses.

Let’s take a look at the average time in trades for each approach, and then normalize the average profit per trade of each approach to a 45-day period.

Here were the average number of calendar days held for the trades in each approach:

As we’d expect, closing trades early for profits or losses results in less time in each trade. As a result, shorter average trade durations lead to more occurrences over time if a new trade is placed immediately after the previous one is closed.

Average P/L Per Trade (Adjusted for Time)

Let’s look at the average profitability of trades for each approach when normalized to a 45-day period. Here’s the calculation:

45-Day Adjusted Average P/L: Average P/L x (45 Days / Average Days in Trade)

SPY Iron Condors: Average Profit/Loss Adjusted for Time

When normalizing the average P/L per trade of each iron condor management approach, we find that there are many better alternatives to managing iron condors than simply holding to expiration (as the previous graphs may have suggested).

Based on these values, the 50-75% profit levels seem to stand out the most.

Commission Impact

Of course, we need to consider the commission impact of each approach. At this point, we’ve identified that the 45-day profit expectancy seems to be consistently high in the 50% and 75% profit-taking combinations. However, with more trade occurrences, commissions can become an issue, especially for an options strategy with four legs.

Let’s take a look at the 45-day expected commissions based on a $1 per contract commission schedule (no ticket/per-trade charge). Here’s the calculation for our commission estimations:

45-Day Commissions = (45 Days / Avg. Days Held) x $1 x 4 Contracts x 2 Trades (Open/Close)

= (45 Days / Avg. Days Held) x $8

As expected, the highest commissions occur in the lower profit/loss approaches, as those combinations generate the highest number of trades.

Average 45-Day P/L – 45-Day Commissions

Let’s look at the average 45-day P/L of each approach after taking out the estimated commissions over each 45-day period:

Iron Condor Commission-Adjusted Profit/Loss

Wow! Before adjusting for commissions, it seemed as if each approach had similar P/L expectancy. However, after taking out the estimated commissions over each 45-day period, it becomes clear that taking profits at 25% may not be the most efficient strategy.

Of course, these figures will vary depending on your particular commission rate, but a $1/contract commission structure with no ticket charge is on the lower end of available commission structures.

In the next section, we’ll examine the 30-delta short option and 16-delta long option iron condor variation.

Results: 30/16 Iron Condors (30-Delta Short, 16-Delta Long)

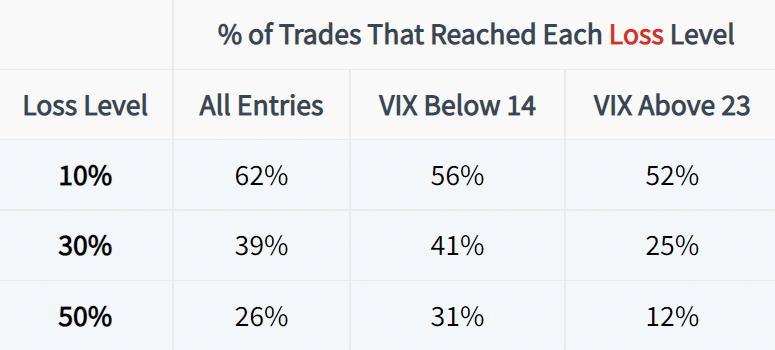
You’ll notice that we’ve omitted the -300% loss level for this particular iron condor setup. We did this because the spread widths are much narrower than the 16-delta short / 5-delta long iron condors. Only 2.5% of trades had loss potential greater than 300% of the credit received. However, 25% of trades had loss potential greater than 250% of the credit received, so we did include the -200% loss level.

Overall, having 12 iron condor management combinations gives us 30,549 trades.

Win Rates: 30-Delta / 16-Delta Iron Condors

Here were the percentages of profitable trades in each iron condor management approach:

Compared to the 16-delta short option and 5-delta long option iron condor setup, the 30-delta / 16-delta iron condors realized lower success rates across the board. However, the 25-50% profit percentage approaches still realized high success rates, despite the iron condors having an approximate 40% probability of expiring worthless.

Let’s see how these win rates stacked up against the required breakeven win rates calculated from the average profits and average losses for each approach.

Win Rates – Breakeven Win Rates

Here are the differences between the realized success rates and required breakeven success rates for each approach:

SPY Iron Condors: Win Rates - Breakeven Success Rates

Completely opposite of the 16-delta iron condors, the 25% profit percentage approaches for the 30-delta iron condors saw the highest margins over the required breakeven success rates. Conversely, the higher profit percentage approaches exceeded the required breakeven win rates by very thin margins.

The data suggests that when the short options are closer to the stock price (closer to ±0.50 delta), closing profitable trades early has been more favorable than closing at higher profit percentages.

Average P/L Per Trade

Let’s take a look at the average profit/loss per trade in each iron condor management approach:

As the Win Rate – Required Breakeven Win Rates would suggest, the 25-50% profit percentage approaches saw the highest average P/L per trade. Interestingly, the -200% loss category experienced higher average P/L than the no-loss approaches. This can be explained by the fact that -200% is near max loss for most of these iron condors. While locking in the maximum loss on a limited-risk spread isn’t very logical in practice, it’s still interesting to see that taking losses slightly lower than the maximum loss enhanced average profitability over the long-term.

90th Percentile Losses

To compare the largest drawdowns of each approach, we’ll examine the 90th percentile losses for each iron condor management combination. The 90th percentile loss tells us the loss level in which 90% of losing trades did not reach. In other words, only 10% of losses were worse than the 90th percentile loss.

As expected, the tighter stop-loss approaches had the shallowest drawdowns of any approach. The -200% loss combinations saw drawdowns very close to the no stop-loss approach of holding trades to expiration, which suggests the trades were closed without much more loss potential (not the most logical approach, but included nonetheless).

Average Time in Trade

As we did before, let’s examine the average time in trades for each approach, and then normalize the average profit per trade of each approach to a 45-day period.

Here were the average number of calendar days held for the trades in each iron condor management approach:

Average P/L Per Trade (Adjusted for Time)

Let’s look at the average profitability of trades for each approach when normalized to a 45-day period. Here’s the calculation for reference:

45-Day Adjusted Average P/L: Average P/L x (45 Days / Average Days in Trade)

When normalizing the average P/L per trade of each iron condor management approach, the 25% profit levels were the clear winners, with the 50% profit levels following close behind. But, as we know, we need to see the percentage of profits given back to commissions before determining the “optimal” management approaches.

Commission Impact

Like we did for the previous iron condor variation, let’s look at the 45-day avg. P/L when adjusted for commissions. Estimated commissions are based on a $1 per contract commission schedule (no ticket/per-trade charge).

First, here are the estimated 45-day commissions for each approach:

Consistent with the findings from the 16-delta iron condors, closing trades earlier (for a profit or loss) generates more overall occurrences in the same period of time. As a result, the commissions were the highest in the 25-50% profit percentage approaches.

Now let’s subtract these commissions from the 45-day P/L expectancy for each approach:

SPY Iron Condors: Commission Adjusted P/L

Opposite of the 16-delta iron condors, the 25-50% profit target combinations had the highest P/L expectancy when adjusted for commissions.

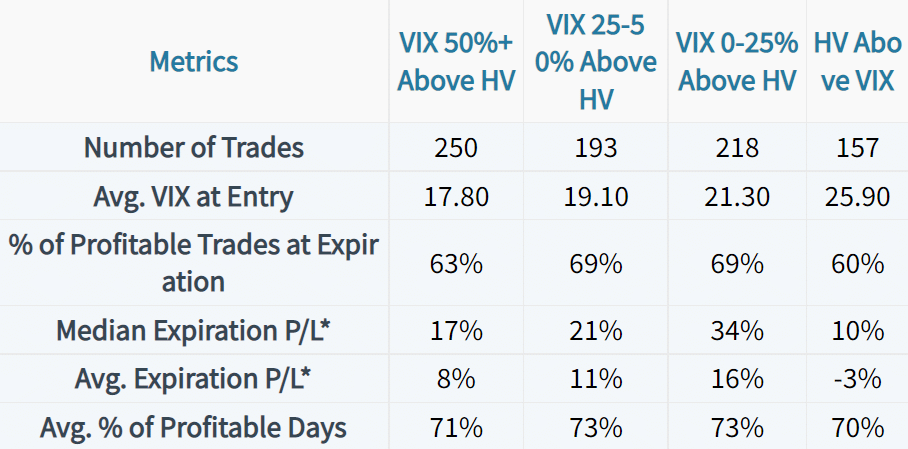
Iron Condor Performance by VIX Level

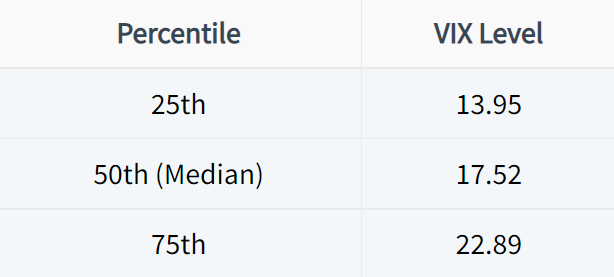
Now that we’ve examined the performance of two iron condor variations with 16 different management combinations, let’s filter the trades into four VIX buckets with an equal number of trades. To accomplish this, we’ll use the 25th, 50th, and 75th percentile of VIX levels over the test period to evenly divide the trades into four volatility buckets.

Here are the four VIX ranges for each bucket:

Low: VIX Below 14

Low-Mid: VIX Between 14 and 17.5

Mid-High: VIX Between 17.5 and 23.5

High: VIX Above 23.5

16-Delta Iron Condors vs. VIX at Entry

Let’s start with success rates:

The results show consistently higher success rates in trades entered when the VIX was below 17.5, with some management combinations realizing a spike in success rates when trades were entered in high implied volatility environments.

How do these success rates stack up against the required breakeven success rates (based on average profits and average losses)?

Win Rates – Breakeven Win Rates

Here’s the difference between the realized success rates and required breakeven success rates for each approach:

In the approaches that did not take losses, the highest margins of success rates over the breakeven success rates occurred in the low IV entries. The approaches that took losses with high profit targets exceeded the required breakeven success rate by the largest margin in the high IV entries.

The findings suggest that when iron condors are sold in high IV environments, the losses tend to be substantial relative to the profits (especially when closing profits early). By taking early losses on losing trades in high IV environments, the losses are kept manageable, while profitable trades drive solid returns on a per-trade basis.

In the next section, we’ll look at profit metrics for the trades in each volatility environment.

Average Profit/Loss Per Trade

Here were the average P/L figures for the iron condors in each approach:

The average P/L metrics tell us that managing losing trades in high IV environments substantially increased the overall profitability of trades, as losses were kept small while profitable trades enjoyed heightened gains.

Additionally, closing profitable trades at lower profit percentages in high IV environments had the worst performance, as profitable trades were cut short while losses were still substantial. The combination of these two things results in a high required win rate to break even, which makes it harder to profit over the long-term.

90th Percentile Losses

To validate previous statements, we’ll examine the 90th percentile losses for the iron condors in each volatility environment. The 90th percentile loss indicates the level that only 10% of trades exceeded. In other words, the 90th percentile loss is rare.

As we can see, the high IV entries saw the worst drawdowns in every single management approach. These loss figures help explain why taking losses in the higher IV entries substantially improved overall profitability per trade.

Average Time in Trade

Here’s the average number of calendar days held for the trades in each approach:

As we’d expect, closing trades early for profits or losses results in less time in each trade.

Average P/L Per Trade (Adjusted for Time)

Let’s look at the average profitability of trades for each approach when normalized to a 45-day period. Here’s the calculation:

45-Day Adjusted Average P/L: Average P/L x (45 Days / Average Days in Trade)

In low volatility environments, closing profitable trades early generated the highest average profitability over 45-day periods. The high IV entries saw the most profitability over 45-day periods in each loss-taking approach. This makes sense, as we know that the largest losses occurred during the high IV entries.

In the final section, we’ll examine how much commissions reduced the performance of each approach.

45-Day Average Profitability (Adjusted for Commissions)

Let’s take a look at the 45-day average P/L of each approach when adjusted for commissions over the same period. Commissions are based on a $1 per contract commission schedule. Here’s the calculation for the adjusted average profitability:

Number of Trades: (45 Days / Avg. Days Held)

Commissions Per Trade: 4 Options x $1 x 2 Transactions (Open/Close) = $8

45-Day Adjusted P/L: 45-Day Avg. P/L – (Commissions Per Trade x Number of Trades)

As we can see here, there are a few approaches with negative P/L expectancy when adjusting for commissions. All of the negative P/L approaches included taking profits at 25% of the maximum profit potential.

The highest P/L expectancies occurred in the loss-taking approaches when entered in high implied volatility environments.

As mentioned earlier, these figures will vary depending on your particular commission rate.

30-Delta Iron Condors vs. VIX at Entry

We’ll finish with the same analysis applied to the 30-delta short option / 16-delta long option iron condor variation!

Let’s start with success rates:

With the short options being much closer to the stock price at entry, closing profitable trades early significantly improved win rates. Furthermore, the larger stop-loss and expiration approaches saw the highest win rates in the lowest and highest VIX entries.

Win Rates – Breakeven Win Rates

Here were the differences between the realized success rates and required breakeven success rates for each approach:

Interestingly, most of the approaches that did not close profitable trades early realized win rates less than the required win rates to break even in the mid-level VIX entries.

The trades with the highest success rates relative to the required breakeven success rates were the high VIX entries, suggesting that selling options closer to the stock price has been beneficial in high volatility environments.

Average P/L Per Trade

Here were the average P/L figures for the iron condors in each approach:

Consistent with the positive Win Rate – Breakeven Success Rate approaches, the iron condor management combinations with the highest average P/L per trade were the high VIX entries.

90th Percentile Losses

How large were the losses in each volatility environment? Here were the 90th percentile losses for each approach:

Similar to the 16-delta iron condors, the largest losses were in the high VIX entries. However, there was less dispersion between losses in each volatility environment, which likely stems from the fact that the 30-delta iron condors have less overall risk relative to the potential reward.

Average Time in Trade

Here’s the average number of calendar days held for the trades in each approach:

As we’d expect, closing trades early for profits or losses results in less time in each trade.

Average P/L Per Trade (Adjusted for Time)

Let’s look at the average profitability of trades for each approach when normalized to a 45-day period. Here’s the calculation:

45-Day Adjusted Average P/L: Average P/L x (45 Days / Average Days in Trade)

By analyzing the 45-day adjusted average P/L for each approach, it becomes very clear that the 30-delta iron condor variation has historically performed very well in high implied volatility environments.

45-Day Average Profitability (Adjusted for Commissions)

Number of Trades: (45 Days / Avg. Days Held)

Commissions Per Trade: 4 Options x $1 x 2 Transactions (Open/Close) = $8

45-Day Adjusted P/L: 45-Day Avg. P/L – (Commissions Per Trade x Number of Trades)

Consistent with previous statements, the high VIX entries were the clear winners in regards to the 30-delta iron condor variation.

Summary

We’ve just made it through a ton of data, so let’s quickly recap the most notable findings from this study!

Iron Condor Variation #1: 16-Delta Short Options & 5-Delta Long Options

In regards to the 16-delta iron condors, each approach appeared to have similar P/L expectancy when accounting for the number of trades that could be squeezed into a 45-day period. However, when adjusted for estimated commissions, the 50-75% profit target approaches stood out the most in terms of P/L expectancy:

When closing profitable trades for 50-75%, the number of overall trades increases since positions are closed faster. This allows traders to “re-center” their iron condor trades sooner, and position themselves in the next expiration cycle. However, the 50-75% profit target combinations do not churn trades as often as the 25% profit target approaches, which results in less commissions and higher expectancy over time.

Implied Volatility & Performance: 16-Delta Short Options & 5-Delta Long Options

When filtering for VIX levels at trade entry, the trades with the highest commission-adjusted P/L expectancy were the loss-management and high profit target combinations with high VIX entries:

Iron Condor Variation #2: 30-Delta Short Options & 16-Delta Long Options

Unlike the 16-delta iron condors, the 30-delta iron condors saw the highest commission-adjusted P/L in the 25-50% profit target approaches. Since the short options are much closer to the stock price, the trades need to be held for much longer to achieve the 75-100% profit levels. Historically, waiting for these profit levels has not been favorable (without filtering for IV at entries).

Implied Volatility & Performance: 30-Delta Short Options & 16-Delta Long Options

After filtering for VIX levels at the entries of all trades, we found that the 30-delta iron condors have historically performed the best when entered in high VIX environments:

No matter the management combination, entering these tighter iron condor trades in high volatility environments has resulted in the most efficient trades in terms of commission- and time-adjusted P/L.

Hopefully, this in-depth study can help guide you towards more informed trading decisions when trading iron condors in equity indices.

Thank you for reading!

Next Lesson

Additional Resources

Iron Condor Options Trading Strategy | tastytrade

Iron Condor FAQs

Are iron condors profitable?

When sold sufficiently out of the money, iron condors have a success rate greater than 50%. However, selling out of the money iron condors comes with greater risk and less profit potential than iron condors sold closer to being in the money.

How much can you lose on an iron condor?

On the short iron condor strategy, the maximum loss is limited to the difference between the strike prices of either spread, multiplied by the contract size, minus the premium received.

Is iron condor safe?

Since the iron condor has defined risk, this options strategy is relatively save when compared to strategies such as the short call and short put.

Option Delta Chain

Calculating Position Delta

Position Delta of Complex Positions

Real Trade Examples

SPY Short Puts

What is Delta Neutral?

Position Delta FAQs

Next Lesson

Option Delta | An Option’s Directional Risk

Delta Hedging for Beginners

How Time Changes Delta

Additional Resources

#1: Lower Capital Requirement

#2: Options Give You The Ability to Use Leverage

#3: Customize Your Strategy

#4: You Can Choose Your Probabilities

#5: Options Can Be Used to Reduce Risk

#6: You Can Combine Options With Stock Investing

#7: You'll Be More In Tune With the Economy

#8: Trading Options is FUN

Final Word

Next Lesson

11 Tips for Options Traders

Calls vs Puts Explained

Options Trading for Beginners

Additional Resources

My First Volatility Mishap

Historical VIX Term Structure Levels

VIX Term Structure and Daily VXX Performance By Year

Implications for Trading Volatility

Next Lesson

VIX Term Structure Explained

CBOE Volatility Index Guide

High Dividend, Low Volatility ETFs

Additional Resources

A Long Calendar Spread’s Profit Drivers

Near-Term vs. Long-Term Option Price Sensitivity

Trade Example #1: Directional Long Calendar Through a Stock Market Correction

Trade Example #2: Long Calendar Spread Profits from an IV Decrease

What’s the Point?

Summary of Main Concepts

Next Lesson

Calendar Spreads Explained

Implied Volatility: The Ultimate Guide

Long Call Calendar Spread Explained

Additional Resources

Study Methodology

Study Results: Taking Profits vs. Taking Losses

Summary of Main Concepts

Next Lesson

Long Strangle Explained

Short Strangle: Rolling Calls

Short Strangle Study: Profit and Loss Analysis

Additional Resources

What Are VXST, VIX, VXV, and VXMT?

Short-Term vs. Long Term Implied Volatility: Calm Market Periods

Short-Term vs. Long Term Implied Volatility: Fearful Market Periods

Summary of Main Concepts

Next Lesson

VIX Term Structure Explained

Here's How VXX Works

High Dividend, Low Volatility ETFs

ETF vs. ETN vs. ETP. vs. ETC: Here’s How They Differ

Additional Resources

What is Historical Volatility?

Implied Volatility vs. Historical Volatility

Study Methodology: Selling Straddles Based on the IV/HV Relationship

Results: Entries Based on the IV/HV Relationship

Summary of Main Concepts

Next Lesson

Implied Volatility Explained

CBOE Volatility Index Guide

High Dividend, Low Volatility ETFs

Additional Resources

Selecting Strike Prices for Stock-Replication Debit Spreads

Selecting Strike Prices for Lower Risk, Higher Return Debit Spreads

Adjusting Strike Prices to Add or Reduce Risk

Keep an Eye on the Short Option’s Price

Summary of Main Concepts

Next Lesson